Tag Surface -- Asymptote Gallery

🔗Asymptote Gallery Tagged by “Surface” #152

🔗animations-fig001

Show animations/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Animation
Tags : #Animation | #Contour | #Surface | #Function (implicit)

import contour3;
import animate;
// settings.tex="pdflatex";
// settings.outformat="pdf";

size(10cm);
currentprojection=orthographic(15,8,10);
animation A;
A.global=false;

typedef real fct3(real,real,real);
fct3 F(real t)
{
  return new real(real x, real y, real z){return x^2+y^2-t*z^2+t-1;};
}

int n=10;
picture pic;
real tmin=0.1, tmax=2;
real step=(tmax-tmin)/n;
draw(box((-5,-5,-5),(5,5,5)));
for (int i=0; i < n; ++i) {
  save();
  draw(surface(contour3(F(tmin+i*step),(-5,-5,-5),(5,5,5),15)),lightblue);
  pic.erase();
  add(pic,bbox(5mm,FillDraw(lightyellow)));
  A.add(pic);
  restore();
}

A.movie();

🔗animations-fig007

Show animations/fig0080.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Animation
Tags : #Graph (3D) | #Function (graphing) | #Animation | #Sphere | #Surface | #Path3 | #Segment

size(16cm);
import graph3;
import animation;
import solids;

settings.render=0;
animation A;

int nbpts=500;
real q=2/5;
real pas=5*2*pi/nbpts;
int angle=3;
real R=3;

real x(real t){return R*cos(q*t)*cos(t);}
real y(real t){return R*cos(q*t)*sin(t);}
real z(real t){return R*sin(q*t);}

triple[] P;
real t=-pi;
for (int i=0; i<nbpts; ++i) {
  t+=pas;
  P.push((x(t),y(t),z(t)));
}

currentprojection=orthographic((0,5,2));
currentlight=(3,3,5);

pen p=rgb(0.1,0.1,0.58);
transform3 t=rotate(angle,(0,0,0),(1,0.25,0.25));

filldraw(box((-R-0.5,-R-0.5),(R+0.5,R+0.5)), p, 3mm+black+miterjoin);

revolution r=sphere(O,R);
draw(surface(r),p);

for (int phi=0; phi<360; phi+=angle) {
  bool[] back,front;
  save();

  for (int i=0; i<nbpts; ++i) {
    P[i]=t*P[i];
    bool test=dot(P[i],currentprojection.camera) > 0;
    front.push(test);
  }

  draw(segment(P,front,operator ..),linewidth(1mm));
  draw(segment(P,!front,operator ..),grey);
  A.add();
  restore();
}

A.movie();

🔗animations-fig008

Show animations/fig0090.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Animation
Tags : #Graph (3D) | #Function (graphing) | #Animation | #Sphere | #Surface | #Path3 | #Segment | #Projection (3D) | #Plane

size(16cm);
import graph3;
import animation;
import solids;

currentlight.background=black;
settings.render=0;
animation A;
A.global=false;

int nbpts=500;
real q=2/5;
real pas=5*2*pi/nbpts;
int angle=4;
real R=0.5;
pen p=rgb(0.1,0.1,0.58);
triple center=(1,1,1);
transform3 T=rotate(angle,center,center+X+0.25*Y+0.3*Z);

real x(real t){return center.x+R*cos(q*t)*cos(t);}
real y(real t){return center.y+R*cos(q*t)*sin(t);}
real z(real t){return center.z+R*sin(q*t);}

currentprojection=orthographic(1,1,1);
currentlight=(0,center.y-0.5,2*(center.z+R));

triple U=(center.x+1.1*R,0,0), V=(0,center.y+1.1*R,0);
path3 xy=plane(U,V,(0,0,0));
path3 xz=rotate(90,X)*xy;
path3 yz=rotate(-90,Y)*xy;

triple[] P;
path3 curve;
real t=-pi;
for (int i=0; i < nbpts; ++i) {
  t+=pas;
  triple M=(x(t),y(t),z(t));
  P.push(M);
  curve = curve..M;
}

curve=curve..cycle;

draw(surface(xy), grey);
draw(surface(xz), grey);
draw(surface(yz), grey);

triple xyc=(center.x,center.y,0);
path3 cle=shift(xyc)*scale3(R)*unitcircle3;
surface scle=surface(cle);
draw(scle, black);
draw(rotate(90,X)*scle, black);
draw(rotate(-90,Y)*scle, black);

draw(surface(sphere(center,R)), p);

triple vcam=1e5*currentprojection.camera-center;
for (int phi=0; phi<360; phi+=angle) {
  bool[] back,front;
  save();

  for (int i=0; i<nbpts; ++i) {
    P[i]=T*P[i];
    bool test=dot(P[i]-center,vcam) > 0;
    front.push(test);
  }

  curve=T*curve;
  draw(segment(P,front,operator ..), paleyellow);
  draw(segment(P,!front,operator ..),0.5*(paleyellow+p));
  draw((planeproject(xy)*curve)^^
       (planeproject(xz)*curve)^^
       (planeproject(yz)*curve), paleyellow);

  A.add();
  restore();
}

A.movie();

🔗graph3-fig001

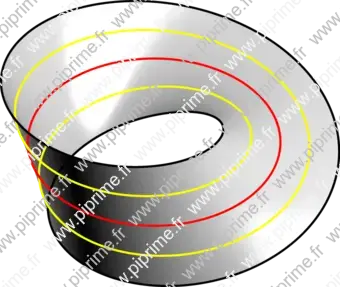
A Möbius strip of half-width $w$ with midcircle of radius

$R$ and at height $z=0$ can be represented parametrically by :

$\backslash begin\{cases\}\%\; x=\backslash left(R+s\backslash times\backslash cos\; \backslash left(\backslash frac\{t\}\{2\}\backslash right)\backslash right)\backslash cos(t)\backslash \backslash \; y=\backslash left(R+s\backslash times\backslash cos\; \backslash left(\backslash frac\{t\}\{2\}\backslash right)\backslash right)\backslash sin(t)\backslash \backslash \; z=s\backslash times\backslash sin\; \backslash left(\backslash frac\{t\}\{2\}\backslash right)\; \backslash end\{cases\}$

for $s$ in $[-w\backslash ,;\backslash ,w]$ and $t$ in $[0\backslash ,;\backslash ,2\backslash pi]$. In this parametrization, the Möbius strip is therefore a cubic surface with equation

$-R2y+x2y+y3-2Rxz-2x2z-2y2z+yz2=0$

Source

Show graph3/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Surface | #Level set (3D)

import graph3;
ngraph=200;
size(12cm,0);
currentprojection=orthographic(-4,-4,5);

real x(real t), y(real t), z(real t);

real R=2;
void xyzset(real s){
  x=new real(real t){return (R+s*cos(t/2))*cos(t);};
  y=new real(real t){return (R+s*cos(t/2))*sin(t);};
  z=new real(real t){return s*sin(t/2);};
}


int n=ngraph;
real w=1;
real s=-w, st=2w/n;
path3 p;
triple[][] ts;
for (int i=0; i <= n; ++i) {
  xyzset(s);
  p=graph(x,y,z,0,2pi);

  ts.push(new triple[] {});
  for (int j=0; j <= ngraph; ++j) {
    ts[i].push(point(p,j));
  }
  s += st;
}

pen[] pens={black, yellow, red, yellow, black};
draw(surface(ts, new bool[][]{}), lightgrey);
for (int i=0; i <= 4; ++i) {
  xyzset(-w+i*w/2);
  draw(graph(x,y,z,0,2pi), 2bp+pens[i]);
}

🔗graph3-fig008

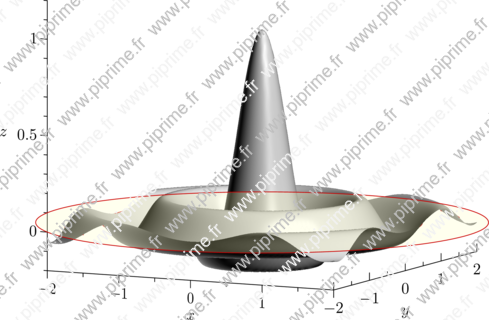
Show graph3/fig0080.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Surface | #Level set (3D) | #Contour | #Function (implicit)

// Adapted from the documentation of Asymptote.
import graph3;
import contour;
texpreamble("\usepackage{icomma}");

size3(12cm, 12cm, 8cm, IgnoreAspect);

real sinc(pair z) {
  real r=2pi*abs(z);
  return r != 0 ? sin(r)/r : 1;
}

limits((-2, -2, -0.2), (2, 2, 1.2));
currentprojection=orthographic(1, -2, 0.5);

xaxis3(rotate(90, X)*"$x$",
       Bounds(Min, Min),
       OutTicks(rotate(90, X)*Label, endlabel=false));

yaxis3("$y$", Bounds(Max, Min), InTicks(Label));
zaxis3("$z$", Bounds(Min, Min), OutTicks());

draw(lift(sinc, contour(sinc, (-2, -2), (2, 2), new real[] {0})), bp+0.8*red);
draw(surface(sinc, (-2, -2), (2, 2), nx=100, Spline), lightgray);

draw(scale3(2*sqrt(2))*unitdisk, paleyellow+opacity(0.25), nolight);
draw(scale3(2*sqrt(2))*unitcircle3, 0.8*red);

🔗graph3-fig009

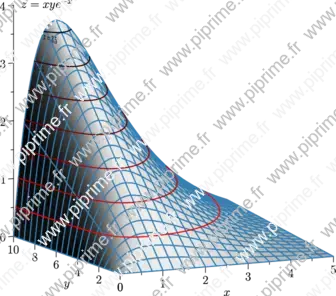
Show graph3/fig0090.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Surface | #Level set (3D) | #Contour | #Function (implicit) | #Palette

size(12cm,0,false);
import graph3;
import contour;
import palette;

texpreamble("\usepackage{icomma}");

real f(pair z) {return z.x*z.y*exp(-z.x);}

currentprojection=orthographic(-2.5,-5,1);

draw(surface(f,(0,0),(5,10),20,Spline),palegray,bp+rgb(0.2,0.5,0.7));

scale(true);

xaxis3(Label("$x$",MidPoint),OutTicks());
yaxis3(Label("$y$",MidPoint),OutTicks(Step=2));
zaxis3(Label("$z=xye^{-x}$",Relative(1),align=2E),Bounds(Min,Max),OutTicks);

real[] datumz={0.5,1,1.5,2,2.5,3,3.5};

Label[] L=sequence(new Label(int i) {
    return YZ()*(Label(format("$z=%g$",datumz[i]),
                       align=2currentprojection.vector()-1.5Z,Relative(1)));
  },datumz.length);

pen fontsize=bp+fontsize(10);
draw(L,lift(f,contour(f,(0,0),(5,10),datumz)),
     palette(datumz,Gradient(fontsize+red,fontsize+black)));

🔗graph3-fig011

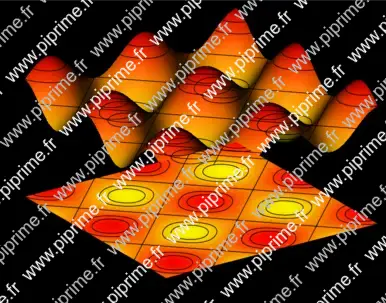
Show graph3/fig0110.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Surface | #Level set (3D) | #Contour | #Function (implicit) | #Palette | #Projection (3D) | #Axis (3D) | #Label (3D) | #Shading (3D) | #Shading

import graph3;
import palette;
import contour;
size(14cm,0);
currentprojection=orthographic(-1,-1.5,0.75);
currentlight=(-1,0,5);

real a=1, b=1;
real f(pair z) { return a*(6+sin(z.x/b)+sin(z.y/b));}
real g(pair z){return f(z)-6a;}

// The axes
limits((0,0,4a),(14,14,8a));
xaxis3(Label("$x$",MidPoint),OutTicks());
yaxis3(Label("$y$",MidPoint),OutTicks(Step=2));
ticklabel relativelabel()
{
  return new string(real x) {return (string)(x-6a);};
}
zaxis3(Label("$z$",Relative(1),align=2E),Bounds(Min,Max),OutTicks(relativelabel()));

// The surface
surface s=surface(f,(0,0),(14,14),100,Spline);

pen[] pens=mean(palette(s.map(zpart),Gradient(yellow,red)));

// Draw the surface
draw(s,pens);
// Project the surface onto the XY plane.
draw(planeproject(unitsquare3)*s,pens,nolight);

// Draw contour for "datumz"
real[] datumz={-1.5, -1, 0, 1, 1.5};
guide[][] pl=contour(g,(0,0),(14,14),datumz);
for (int i=0; i < pl.length; ++i)
  for (int j=0; j < pl[i].length; ++j)
    draw(path3(pl[i][j]));

// Draw the contours on the surface
draw(lift(f,pl));

if(!is3D())
  shipout(bbox(3mm,Fill(black)));

🔗graph3-fig012

Show graph3/fig0120.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Projection (3D) | #Shading (3D)

import graph3;
import palette;

real sinc(real x){return x != 0 ? sin(x)/x : 1;}

real f(pair z){
  real value = (sinc(pi*z.x)*sinc(pi*z.y))**2;
  return value^0.25;
}

currentprojection=orthographic(0,0,1);

size(10cm,0);

surface s=surface(f,(-5,-5),(5,5),100,Spline);
s.colors(palette(s.map(zpart),Gradient((int)2^11 ... new pen[]{black,white})));

draw(planeproject(unitsquare3)*s,nolight);

🔗graph3-fig013

From TeXgraph exemples.

Show graph3/fig0130.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D)

settings.render=0;
import graph3;
import palette;
size(10cm,0);
currentprojection=orthographic(2,-2,2.5);

real f(pair z) {
  real u=z.x, v=z.y;
  return (u/2+v)/(2+cos(u/2)*sin(v));
}

surface s=surface(f,(0,0),(14,14),150,Spline);
draw(s,mean(palette(s.map(zpart),Gradient(yellow,red))));

if(!is3D())
  shipout(bbox(3mm,Fill(black)));

🔗graph3-fig014

From TeXgraph exemples.

Show graph3/fig0140.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D)

settings.render=0;
import graph3;
import palette;
size(15cm,0);
currentprojection=orthographic(2,-2,2.5);

real f(pair z) {
  real u=z.x, v=z.y;
  return (u/2+v)/(2+cos(u/2)*sin(v));
}

surface s=surface(f,(0,0),(14,14),50,Spline);
s.colors(palette(s.map(zpart),Gradient(yellow,red)));

draw(s);

if(!is3D())
  shipout(bbox(3mm,Fill(black)));

🔗graph3-fig015

Show graph3/fig0150.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D) | #Array | #Spherical harmonics

settings.render=0;
import graph3;
size(15cm);

currentprojection=orthographic(4,2,4);

real r(real Theta, real Phi){return 1+0.5*(sin(2*Theta)*sin(2*Phi))^2;}
triple f(pair z) {return r(z.x,z.y)*expi(z.x,z.y);}

pen[] pens(triple[] z)
{
  return sequence(new pen(int i) {
      real a=abs(z[i]);
      return a < 1+1e-3 ? black : interp(blue, red, 2*(a-1));
    },z.length);
}

surface s=surface(f,(0,0),(pi,2pi),100,Spline);
// Interpolate the corners, and coloring each patch with one color
// produce some artefacts
draw(s,pens(s.cornermean()));

if(!is3D())
  shipout(bbox(3mm,Fill(black)));

🔗graph3-fig016

Show graph3/fig0160.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D) | #Array | #Spherical harmonics

settings.render=0;
import graph3;
size(15cm);

currentprojection=orthographic(4,2,4);

real r(real Theta, real Phi){return 1+0.5*(sin(2*Theta)*sin(2*Phi))^2;}
triple f(pair z) {return r(z.x,z.y)*expi(z.x,z.y);}

pen[][] pens(triple[][] z)
{
  pen[][] p=new pen[z.length][];
  for(int i=0; i < z.length; ++i) {
    triple[] zi=z[i];
    p[i]=sequence(new pen(int j) {
	real a=abs(zi[j]);
	return a < 1+1e-3 ? black : interp(blue, red, 2*(a-1));},
      zi.length);
  }
  return p;
}

surface s=surface(f,(0,0),(pi,2pi),100,Spline);
// Here we interpolate the pens, this looks smoother, with fewer artifacts
draw(s,mean(pens(s.corners())));

if(!is3D())
  shipout(bbox(3mm,Fill(black)));

🔗graph3-fig017

Show graph3/fig0170.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D) | #Array | #Spherical harmonics

import graph3;
size(16cm, 0);

currentprojection=orthographic(4, 2, 4);

real r(real Theta, real Phi){return 1+0.5*(sin(2*Theta)*sin(2*Phi))^2;}
triple f(pair z) {return r(z.x, z.y)*expi(z.x, z.y);}

pen[][] pens(triple[][] z)
{
  pen[][] p=new pen[z.length][];
  for(int i=0; i < z.length; ++i) {
    triple[] zi=z[i];
    p[i]=sequence(new pen(int j) {
    real a=abs(zi[j]);

    return a < 1+1e-3 ? black : interp(blue, red, 2*(a-1));}, zi.length);
  }

  return p;
}

surface s=surface(f, (0, 0), (pi, 2pi), 100, Spline);
// Here we determine the colors of vertexes (vertex shading).
// Since the PRC output format does not support vertex shading of Bezier surfaces, PRC patches
// are shaded with the mean of the four vertex colors.
s.colors(pens(s.corners()));
draw(s);

if(!is3D())
  shipout(bbox(1mm, Fill(black)));

🔗graph3-fig018

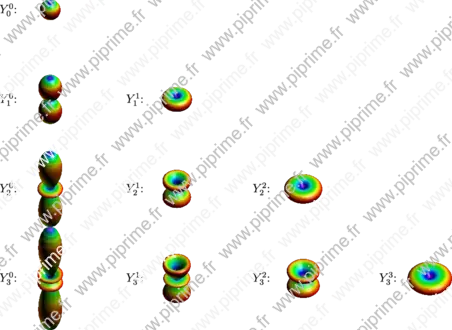
The spherical harmonics $Y\_l^m(\backslash theta,\backslash varphi)$ are the angular portion of the solution to Laplace's equation in spherical coordinates where azimuthal symmetry is not present.

The spherical harmonics are defined by:

$Y\_l^m(\backslash theta,\backslash varphi)=\backslash sqrt\{\backslash frac\{2l+1\}\{4\backslash pi\}\backslash frac\{(l-m)!\}\{(l+m)!\}\}P\_\{l\}^\{m\}(\backslash cos\backslash theta)e^\{im\backslash varphi\}$

where $m=-l,\backslash ,-(l-1),\backslash ,\backslash ldots,\backslash ,0,\backslash ,\backslash ldots,\backslash ,l-1,\backslash ,l$ and $P\_l^m$ is the Legendre polynomial.

Source

Show graph3/fig0180.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Palette | #Surface | #Shading (3D) | #Spherical harmonics

import palette;
import math;
import graph3;

typedef real fct(real);
typedef pair zfct2(real,real);
typedef real fct2(real,real);

real binomial(real n, real k)
{
  return gamma(n+1)/(gamma(n-k+1)*gamma(k+1));
}

real factorial(real n) {
  return gamma(n+1);
}

real[] pdiff(real[] p)
{ // p(x)=p[0]+p[1]x+...p[n]x^n
  // retourne la dérivée de p
  real[] dif;
  for (int i : p.keys) {
    if(i != 0) dif.push(i*p[i]);
  }
  return dif;
}

real[] pdiff(real[] p, int n)
{ // p(x)=p[0]+p[1]x+...p[n]x^n
  // dérivée n-ième de p
  real[] dif={0};
  if(n >= p.length) return dif;
  dif=p;
  for (int i=0; i < n; ++i)
    dif=pdiff(dif);
  return dif;
}

fct operator *(real y, fct f)
{
  return new real(real x){return y*f(x);};
}

zfct2 operator +(zfct2 f, zfct2 g)
{// Défini f+g
  return new pair(real t, real p){return f(t,p)+g(t,p);};
}

zfct2 operator -(zfct2 f, zfct2 g)
{// Défini f-g
  return new pair(real t, real p){return f(t,p)-g(t,p);};
}

zfct2 operator /(zfct2 f, real x)
{// Défini f/x
  return new pair(real t, real p){return f(t,p)/x;};
}

zfct2 operator *(real x,zfct2 f)
{// Défini x*f
  return new pair(real t, real p){return x*f(t,p);};
}

fct fct(real[] p)
{ // convertit le tableau des coefs du poly p en fonction polynôme
  return new real(real x){
    real y=0;
    for (int i : p.keys) {
      y += p[i]*x^i;
    }
    return y;
  };
}

real C(int l, int m)
{
  if(m < 0) return 1/C(l,-m);
  real OC=1;
  int d=l-m, s=l+m;
  for (int i=d+1; i <=s ; ++i) OC *= i;
  return 1/OC;
}

int csphase=-1;
fct P(int l, int m)
{ // Polynôme de Legendre associé
  // http://mathworld.wolfram.com/LegendrePolynomial.html
  if(m < 0) return (-1)^(-m)*C(l,-m)*P(l,-m);
  real[] xl2;
  for (int k=0; k <= l; ++k) {
    xl2.push((-1)^(l-k)*binomial(l,k));
    if(k != l) xl2.push(0);
  }
  fct dxl2=fct(pdiff(xl2,l+m));
  return new real(real x){
    return (csphase)^m/(2^l*factorial(l))*(1-x^2)^(m/2)*dxl2(x);
  };
}

zfct2 Y(int l, int m)
{// http://fr.wikipedia.org/wiki/Harmonique_sph%C3%A9rique#Expression_des_harmoniques_sph.C3.A9riques_normalis.C3.A9es
  return new pair(real theta, real phi) {
    return sqrt((2*l+1)*C(l,m)/(4*pi))*P(l,m)(cos(theta))*expi(m*phi);
  };
}

real xyabs(triple z){return abs(xypart(z));}

size(16cm);
currentprojection=orthographic(0,1,1);

zfct2 Ylm;

triple F(pair z)
{
  //   real r=0.75+dot(0.25*I,Ylm(z.x,z.y));
  //   return r*expi(z.x,z.y);
  real r=abs(Ylm(z.x,z.y))^2;
  return r*expi(z.x,z.y);
}

int nb=4;
for (int l=0; l < nb; ++l) {
  for (int m=0; m <= l; ++m) {
    Ylm=Y(l,m);

    surface s=surface(F,(0,0),(pi,2pi),60);
    s.colors(palette(s.map(xyabs),Rainbow()));

    triple v=(-m,0,-l);
    draw(shift(v)*s);
    label("$Y_"+ string(l) + "^" + string(m) + "$:",shift(X/3)*v);
  }
}

🔗solids-fig001

Show solids/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Light (3D) | #Draw (3D) | #Surface | #Sphere | #Revolution | #Projection (3D)

import solids;
import three;
currentprojection=orthographic(1,2,2);

size(6cm,0);

material m = material(
  diffusepen=yellow,
  emissivepen=black,
  specularpen=orange,
  shininess=0.25,
  metallic=0.5,
  fresnel0=0.07
);

draw(surface(sphere(1)), m);

🔗solids-fig002

Show solids/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Light (3D) | #Draw (3D) | #Surface | #Sphere | #Revolution | #Projection (3D)

import solids;
currentlight=light(paleyellow, specularfactor=3, (2,4,6));

size(6cm,0);
draw(sphere(1,n=4*nslice), linewidth(bp), m=10);
draw(surface(sphere(1,n=4*nslice)), orange);

🔗solids-fig003

Show solids/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Revolution | #Projection (3D) | #Draw (3D) | #Surface | #Sphere | #Wire frame | #Line type

// Author: John Bowman.
size(6cm, 0);
import solids;
currentprojection=orthographic(0, 10, 5);

nslice=4*nslice;

revolution r=sphere(O, 1);
draw(surface(r), lightgrey+opacity(0.75));

skeleton s;
r.transverse(s, reltime(r.g, 0.6), currentprojection);
r.transverse(s, reltime(r.g, 0.5), currentprojection);
draw(s.transverse.back, linetype("8 8", 8));
draw(s.transverse.front);

r.longitudinal(s, currentprojection);
draw(s.longitudinal.front);
draw(s.longitudinal.back, linetype("8 8", 8));

🔗solids-fig004

Show solids/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Transform (3D) | #Revolution | #Surface | #Sphere | #Projection (3D)

import solids;
size(6cm,0);

currentprojection=orthographic(100,150,30);

real r=1;

skeleton s;
revolution sph=sphere(O,r);
draw(surface(sph), palegray);

path3 cle=rotate(90,X)*scale3(r)*unitcircle3;

triple cam=unit(currentprojection.camera);
real a=degrees(xypart(cam),false)-90;
real b=-sgn(cam.z)*aCos(sqrt(cam.x^2+cam.y^2)/abs(cam));
cle=rotate(b,cross(Z,cam))*rotate(a,Z)*cle;
draw(cle,4pt+red);

🔗solids-fig005

Show solids/fig0050.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Projection (3D) | #Solid | #Transform (3D) | #Revolution | #Surface | #Sphere | #Light (3D)

import solids;
size(6cm,0);
currentlight=light(diffuse=yellow, specular=blue, specularfactor=5, (5,-5,10));
// currentprojection=orthographic(100,100,30);
real r=2;

skeleton s;
revolution sph=sphere(O,r);
draw(surface(sph),red);

triple cam=unit(currentprojection.camera);
revolution cle=revolution(O,r*(rotate(90,Z)*cam),cam);
draw(cle, 8pt+black);

🔗solids-fig007

Show solids/fig0070.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Surface | #Sphere | #Draw (3D)

import solids;
size(6cm,0);
currentprojection=orthographic(1,2,2);

surface s=surface(sphere(1,n=10));

material m = material(
  diffusepen = 0.8*red,
  emissivepen= yellow,
  specularpen= red
);

material[] p={m, red, 0.8*(red+blue) , green, 0.8*blue};
p.cyclic=true;

draw(s,p);

🔗solids-fig008

Show solids/fig0080.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Plane | #Shading (3D) | #Transform (3D) | #Projection (3D) | #Surface | #Palette

import solids;
import palette;
size(14cm,0);
currentlight=light(
  gray(0.4),
  specularfactor=3,
  (-0.5,-0.25,0.45),
  (0.5,-0.5,0.5),(0.5,0.5,0.75)
);

nslice=4*nslice;
surface s=surface(sphere(O,1));
draw(s,lightgrey);

path3 pl=plane((1,0,0),(0,1,0),(0,0,-1));
surface pls=shift(3,3,-1e-3)*scale(-6,-6,1)*surface(pl);
draw(pls,0.7*red);

real dist(triple z){return abs(z-Z);}

surface shade;
for (int i=0; i < currentlight.position.length; ++i) {
  shade=planeproject(pl,currentlight.position[i])*s;
  draw(shade,mean(palette((shade.map(dist)),
                          Gradient(black,gray(0.6)))),
       nolight);
}

🔗solids-fig009

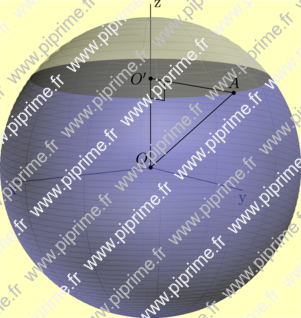
Show solids/fig0090.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Arc (3D) | #Markers | #Revolution | #Surface | #Sphere

size(8cm, 0);
import solids;
import graph3;

//Draw 3D right angle (MA, MB)
void drawrightangle(picture pic=currentpicture,
                    triple M, triple A, triple B,
                    real radius=0,
                    pen p=currentpen,
                    pen fillpen=nullpen,
                    projection P=currentprojection)
{
  p=linejoin(0)+linecap(0)+p;
  if (radius==0) radius=arrowfactor*sqrt(2);
  transform3 T=shift(-M);
  triple OA=radius/sqrt(2)*unit(T*A),
    OB=radius/sqrt(2)*unit(T*B),
    OC=OA+OB;
  path3 tp=OA--OC--OB;
  picture tpic;
  draw(tpic, tp, p=p);
  if (fillpen!=nullpen) draw(tpic, surface(O--tp--cycle), fillpen);
  add(pic, tpic, M);
}

currentprojection=orthographic(10, 15, 3);

real r=10, h=6; // r=sphere radius; h=altitude section
triple Op=(0, 0, h);

limits((0, 0, 0), 1.1*(r, r, r));
axes3("x", "y", "z");

real rs=sqrt(r^2-h^2); // section radius
real ch=180*acos(h/r)/pi;
path3 arcD=Arc(O, r, 180, 0, ch, 0, Y, 50);

revolution sphereD=revolution(O, arcD, Z);
draw(surface(sphereD), opacity(0.5)+lightblue);
draw(shift(0, 0, h)*scale3(rs)*surface(unitcircle3), opacity(0.5));

path3 arcU=Arc(O, r, ch, 0, 0, 0, Y, 10);
revolution sphereU=revolution(O, arcU, Z);
draw(surface(sphereU), opacity(0.33)+lightgrey);

// right triangle OO'A
triple A=rotate(100, Z)*(rs, 0, h);
dot("$O$", O, NW);
dot("$O'$", Op, W);
dot("$A$", A, N);
draw(A--O--Op--A);
drawrightangle(Op, O, A);

if(!is3D())
  shipout(format="pdf", bbox(Fill(paleyellow)));

🔗solids-fig010

Show solids/fig0100.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Revolution | #Wire frame | #Surface

unitsize(1cm);
import solids;

currentprojection=orthographic(0, 100, 25);

real r=4, h=7;
triple O=(0, 0, 0);
triple Oprime=(0, 0, 3);
triple pS=(0, 0, h);
triple pA=(r*sqrt(2)/2, r*sqrt(2)/2, 0);
revolution rC=cone(O, r, h, axis=Z, n=1);

draw(surface(rC), blue+opacity(0.5));

skeleton s;
real tOprime=abs(Oprime)/h;
rC.transverse(s, reltime(rC.g, tOprime), currentprojection);
triple pAprime=relpoint(pA--pS, tOprime);
draw(s.transverse.back, dashed);
draw(s.transverse.front);

label("$S$", pS, N);
dot(Label("$O$", align=SE), O);
dot(Label("$O'$", align=SE), Oprime);
dot(Label("$A$", align=Z), pA);
dot(Label("$A'$", align=Z), pAprime);

draw(pS--O^^O--pA^^Oprime--pAprime, dashed);

🔗solids-fig014

Author : Jens Schwaiger.
With its pleasant authorization.

Show solids/fig0140.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Polyhedron | #Surface

size(10cm,0);
import bsp;

currentprojection=perspective(10,3,-2);
guide achteck=polygon(8);
real lge=length(point(achteck,1)-point(achteck,0));
int n=8;
face[] faces;
guide3[] sq;
guide3[] tr;
triple a,b,c,d;

a=(point(achteck,0).x,point(achteck,0).y,-lge/2);
b=(point(achteck,1).x,point(achteck,1).y,-lge/2);
c=(point(achteck,1).x,point(achteck,1).y,lge/2);
d=(point(achteck,0).x,point(achteck,0).y,lge/2);

sq[0]=a--b--c--d--cycle;
for(int i=1;i<n;i=i+1) sq[i]=rotate(45*i,Z)*sq[0];
for(int i=0;i<3;i=i+1) sq[n+i]=rotate(90,Y)*sq[i];
for(int i=4;i<7;i=i+1) sq[n-1+i]=rotate(90,Y)*sq[i];
for(int i=2;i<3;i=i+1) sq[12+i]=rotate(90,X)*sq[i];
sq[14]=rotate(90,X)*sq[2];
sq[15]=rotate(90,X)*sq[4];
sq[16]=rotate(90,X)*sq[6];
sq[17]=rotate(90,X)*sq[0];

tr[0]=point(sq[2],3)--point(sq[2],2)--point(sq[14],1)--cycle;
for(int i=1;i<4;i=i+1) tr[i]=rotate(90*i,Z)*tr[0];
tr[4]=reverse(point(sq[2],0)--point(sq[2],1)--point(sq[9],2)--cycle);
for(int i=5;i<8;i=i+1) tr[i]=rotate(90*(i-4),Z)*tr[4];

real hgtsq=3;
triple[][][] pyrsq=new triple[18][4][3];
path3[] pyrsqfc=new path3[4*18];
int nofface=0;
for(int i=0;i<18;i=i+1){
  triple cog=0.5(point(sq[i],0)+point(sq[i],2));
  triple sp=cog+
    hgtsq*unit(cross(point(sq[i],1)-point(sq[i],0),point(sq[i],3)-point(sq[i],0)));
  for(int j=0;j<3;j=j+1){
    pyrsq[i][j][0]=point(sq[i],j);
    pyrsq[i][j][1]=point(sq[i],j+1);
    pyrsq[i][j][2]=sp;
    pyrsqfc[nofface]=pyrsq[i][j][0]--pyrsq[i][j][1]--pyrsq[i][j][2]--cycle;
    nofface=nofface+1;
  }
  pyrsq[i][3][0]=point(sq[i],3);
  pyrsq[i][3][1]=point(sq[i],0);
  pyrsq[i][3][2]=sp;
  pyrsqfc[nofface]=pyrsq[i][3][0]--pyrsq[i][3][1]--pyrsq[i][3][2]--cycle;
  nofface=nofface+1;
 }

for(int i=0;i<18*4;i=i+1) faces.push(pyrsqfc[i]);
for(int i=0;i<18*4;i=i+1) filldraw(faces[i],project(pyrsqfc[i]),yellow,black+2.5bp);

path3[] pyrtrfc=new path3[3*8];
real hgttr=2;
int nuoftr=0;

for(int i=0;i<8;i=i+1){
  triple cog=(1/3)*(point(tr[i],0)+point(tr[i],1)+point(tr[i],2));
  triple sp=cog+hgttr*unit(cross(point(tr[i],1)-point(tr[i],0),point(tr[i],2)-point(tr[i],0)));
  pyrtrfc[nuoftr]=point(tr[i],0)--point(tr[i],1)--sp--cycle;
  pyrtrfc[nuoftr+1]=point(tr[i],1)--point(tr[i],2)--sp--cycle;
  pyrtrfc[nuoftr+2]=point(tr[i],2)--point(tr[i],0)--sp--cycle;
  nuoftr=nuoftr+3;
 }

for(int j=0;j<24;j=j+1) faces.push(pyrtrfc[j]);
for(int j=0;j<24;j=j+1) filldraw(faces[4*18+j],project(pyrtrfc[j]),orange+yellow,black+2bp);

add(faces);
shipout(defaultfilename,bbox(0.2cm,black,RadialShade(paleblue,darkblue)));

🔗solids-fig015

Author : Jens Schwaiger.
With its pleasant authorization.

Show solids/fig0150.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Solids.asy
Tags : #Solid | #Polyhedron | #Surface

// PRC/OpenGL version

size(10cm,0);
import graph3;

currentprojection=orthographic(10,3,-2);
// currentlight=nolight;

guide achteck=polygon(8);
real lge=length(point(achteck,1)-point(achteck,0));
int n=8;
guide3[] sq;
guide3[] tr;
triple a,b,c,d;

a=(point(achteck,0).x,point(achteck,0).y,-lge/2);
b=(point(achteck,1).x,point(achteck,1).y,-lge/2);
c=(point(achteck,1).x,point(achteck,1).y,lge/2);
d=(point(achteck,0).x,point(achteck,0).y,lge/2);

sq[0]=a--b--c--d--cycle;
for(int i=1;i<n;i=i+1) sq[i]=rotate(45*i,Z)*sq[0];
for(int i=0;i<3;i=i+1) sq[n+i]=rotate(90,Y)*sq[i];
for(int i=4;i<7;i=i+1) sq[n-1+i]=rotate(90,Y)*sq[i];
for(int i=2;i<3;i=i+1) sq[12+i]=rotate(90,X)*sq[i];
sq[14]=rotate(90,X)*sq[2];
sq[15]=rotate(90,X)*sq[4];
sq[16]=rotate(90,X)*sq[6];
sq[17]=rotate(90,X)*sq[0];

tr[0]=point(sq[2],3)--point(sq[2],2)--point(sq[14],1)--cycle;
for(int i=1;i<4;i=i+1) tr[i]=rotate(90*i,Z)*tr[0];
tr[4]=reverse(point(sq[2],0)--point(sq[2],1)--point(sq[9],2)--cycle);
for(int i=5;i<8;i=i+1) tr[i]=rotate(90*(i-4),Z)*tr[4];

real hgtsq=3;
triple[][][] pyrsq=new triple[18][4][3];
path3[] pyrsqfc=new path3[4*18];
int nofface=0;
for(int i=0;i<18;i=i+1){
  triple cog=0.5(point(sq[i],0)+point(sq[i],2));
  triple sp=cog+
    hgtsq*unit(cross(point(sq[i],1)-point(sq[i],0),point(sq[i],3)-point(sq[i],0)));
  for(int j=0;j<3;j=j+1){
    pyrsq[i][j][0]=point(sq[i],j);
    pyrsq[i][j][1]=point(sq[i],j+1);
    pyrsq[i][j][2]=sp;
    pyrsqfc[nofface]=pyrsq[i][j][0]--pyrsq[i][j][1]--pyrsq[i][j][2]--cycle;
    nofface=nofface+1;
  }
  pyrsq[i][3][0]=point(sq[i],3);
  pyrsq[i][3][1]=point(sq[i],0);
  pyrsq[i][3][2]=sp;
  pyrsqfc[nofface]=pyrsq[i][3][0]--pyrsq[i][3][1]--pyrsq[i][3][2]--cycle;
  nofface=nofface+1;
 }

for(int i=0;i<18*4;i=i+1)
  draw(surface(pyrsqfc[i]),yellow,black+2.5bp);

path3[] pyrtrfc=new path3[3*8];
real hgttr=2;
int nuoftr=0;

for(int i=0;i<8;i=i+1){
  triple cog=(1/3)*(point(tr[i],0)+point(tr[i],1)+point(tr[i],2));
  triple sp=cog+hgttr*unit(cross(point(tr[i],1)-point(tr[i],0),point(tr[i],2)-point(tr[i],0)));
  pyrtrfc[nuoftr]=point(tr[i],0)--point(tr[i],1)--sp--cycle;
  pyrtrfc[nuoftr+1]=point(tr[i],1)--point(tr[i],2)--sp--cycle;
  pyrtrfc[nuoftr+2]=point(tr[i],2)--point(tr[i],0)--sp--cycle;
  nuoftr=nuoftr+3;
 }

for(int j=0;j<24;j=j+1)
  draw(surface(pyrtrfc[j]),orange+yellow,black+2bp);

🔗three-fig001

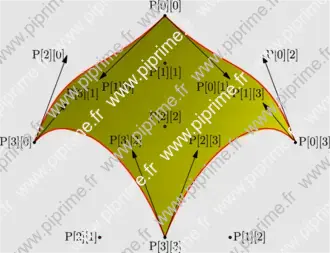
Show three/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Three.asy
Tags : #Direction (3D) | #Patch | #Path3 | #Surface | #Triple

import three;

size(12cm);
currentprojection=orthographic(1,1,1.5);
currentlight=(1,0,1);

triple P00=-X-Y+0.5*Z, P03=-X+Y, P33=X+Y, P30=X-Y;

triple[][] P={
  {P00,P00+(-0.5,0.5,-1),P03+(0,-0.5,1),P03},
  {P00+(0.5,-0.5,-1),(-0.5,-0.5,0.5),(-0.5,0.5,-1.5),P03+(0.5,0,1)},
  {P30+(-0.5,0,1),(0.5,-0.5,-1.5),(0.5,0.5,1),P33+(-0.5,0,1)},
  {P30,P30+(0,0.5,1),P33+(0,-0.5,1),P33}
};

surface s=surface(patch(P));
draw(s,15,15,yellow,meshpen=grey);
draw(sequence(new path3(int i){
      return s.s[i].external();},s.s.length), bp+red);

dot("P[0][0]",P[0][0], align=N, black);
dot("P[0][3]",P[0][3], black);
dot("P[3][3]",P[3][3], align=S, black);
dot("P[3][0]",P[3][0], align=W, black);

draw(Label("P[0][1]",align=SW,EndPoint),P[0][0]--P[0][1], Arrow3);
draw(Label("P[1][0]",align=SE,EndPoint),P[0][0]--P[1][0], Arrow3);

draw(Label("P[0][2]",align=E,EndPoint),P[0][3]--P[0][2], Arrow3);
draw(Label("P[1][3]",EndPoint),P[0][3]--P[1][3], Arrow3);

draw(Label("P[2][3]",EndPoint),P[3][3]--P[2][3], Arrow3);
draw(Label("P[3][2]",EndPoint),P[3][3]--P[3][2], Arrow3);

draw(Label("P[3][1]",EndPoint),P[3][0]--P[3][1], Arrow3);
draw(Label("P[2][0]", align=W,EndPoint),P[3][0]--P[2][0], Arrow3);


dot("P[1][1]",P[1][1], align=S);
dot("P[1][2]",P[1][2], align=E);
dot("P[2][2]",P[2][2], align=N);
dot("P[2][1]",P[2][1], align=W);

for (int i=0; i < s.s.length; ++i)
  dot(s.s[i].internal(), bp+red);

if(!is3D())
  shipout(bbox(Fill(lightgrey)));

🔗three-fig002

Show three/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Three.asy
Tags : #Shading (3D) | #Palette | #Patch | #Path3 | #Surface | #Triple

import three;
import palette;

size(12cm);
currentprojection=orthographic(1,1,1.5);
currentlight=(1,0,1);

triple P00=-X-Y+0.5*Z, P03=-X+Y, P33=X+Y, P30=X-Y;

triple[][] P={
  {P00,P00+(-0.5,0.5,-1),P03+(0,-0.5,1),P03},
  {P00+(0.5,-0.5,-1),(-0.5,-0.5,0.5),(-0.5,0.5,-1.5),P03+(0.5,0,1)},
  {P30+(-0.5,0,1),(0.5,-0.5,-1.5),(0.5,0.5,1),P33+(-0.5,0,1)},
  {P30,P30+(0,0.5,1),P33+(0,-0.5,1),P33}
};

surface s=surface(patch(P));
s.colors(palette(s.map(zpart),Gradient(yellow,red)));
// s.colors(palette(s.map(zpart),Rainbow()));

draw(s);
draw(sequence(new path3(int i){
      return s.s[i].external();},s.s.length), bp+orange);


if(!is3D())
  shipout(bbox(Fill(lightgrey)));

🔗three-fig003

Show three/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Three.asy
Tags : #Shading (3D) | #Palette | #Patch | #Path3 | #Surface | #Triple

import three;

size(10cm);
currentlight=(0,0,1);

surface sf=surface(patch(P=new triple[][] {
      {(0,0,0),(1,0,0),(1,0,0),(2,0,0)},
      {(0,1,0),(1,0,1),(1,0,1),(2,1,0)},
      {(0,1,0),(1,0,-1),(1,0,-1),(2,1,0)},
      {(0,2,0),(1,2,0),(1,2,0),(2,2,0)}
    }));

draw(sf,surfacepen=yellow);
draw(sf.s[0].vequals(0.5),squarecap+2bp+blue,currentlight);
draw(sf.s[0].uequals(0.5),squarecap+2bp+red,currentlight);

This page was generated by the Asymptote Exemples Builder
Last modified the 2025-04-20 12:26:19
©2011 Philippe Ivaldi