Tag Level set (3D) -- Asymptote Gallery

🔗Asymptote Gallery Tagged by “Level set (3D)” #159

🔗graph3-fig001

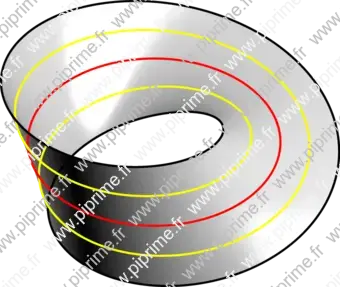
Figure graph3 001 Generated with Asymptote

A MÃ¶bius strip of half-width $w$ with midcircle of radius

$R$ and at height $z=0$ can be represented parametrically byÂ :

\begin{cases}%
x=\left(R+s\times\cos \left(\frac{t}{2}\right)\right)\cos(t)\\
y=\left(R+s\times\cos \left(\frac{t}{2}\right)\right)\sin(t)\\
z=s\times\sin \left(\frac{t}{2}\right)
\end{cases}

for $s$ in $[-w\,;\,w]$ and $t$ in $[0\,;\,2\pi]$ . In this parametrization, the MÃ¶bius strip is therefore a cubic surface with equation

$-R$ ^2y+x2y+y^3-2Rxz-2x2z-2y^2z+yz2=0

Source

Show graph3/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Examples 3D | Graph3.asy
Tags : #Graph (3D) | #Surface | #Level set (3D)

import graph3;
ngraph=200;
size(12cm,0);
currentprojection=orthographic(-4,-4,5);

real x(real t), y(real t), z(real t);

real R=2;
void xyzset(real s){
  x=new real(real t){return (R+s*cos(t/2))*cos(t);};
  y=new real(real t){return (R+s*cos(t/2))*sin(t);};
  z=new real(real t){return s*sin(t/2);};
}


int n=ngraph;
real w=1;
real s=-w, st=2w/n;
path3 p;
triple[][] ts;
for (int i=0; i <= n; ++i) {
  xyzset(s);
  p=graph(x,y,z,0,2pi);

  ts.push(new triple[] {});
  for (int j=0; j <= ngraph; ++j) {
    ts[i].push(point(p,j));
  }
  s += st;
}

pen[] pens={black, yellow, red, yellow, black};
draw(surface(ts, new bool[][]{}), lightgrey);
for (int i=0; i <= 4; ++i) {
  xyzset(-w+i*w/2);
  draw(graph(x,y,z,0,2pi), 2bp+pens[i]);
}

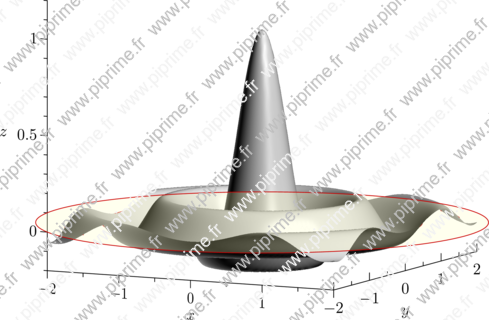
🔗graph3-fig008

Figure graph3 008 Generated with Asymptote

// Adapted from the documentation of Asymptote.
import graph3;
import contour;
texpreamble("\usepackage{icomma}");

size3(12cm, 12cm, 8cm, IgnoreAspect);

real sinc(pair z) {
  real r=2pi*abs(z);
  return r != 0 ? sin(r)/r : 1;
}

limits((-2, -2, -0.2), (2, 2, 1.2));
currentprojection=orthographic(1, -2, 0.5);

xaxis3(rotate(90, X)*"$x$",
       Bounds(Min, Min),
       OutTicks(rotate(90, X)*Label, endlabel=false));

yaxis3("$y$", Bounds(Max, Min), InTicks(Label));
zaxis3("$z$", Bounds(Min, Min), OutTicks());

draw(lift(sinc, contour(sinc, (-2, -2), (2, 2), new real[] {0})), bp+0.8*red);
draw(surface(sinc, (-2, -2), (2, 2), nx=100, Spline), lightgray);

draw(scale3(2*sqrt(2))*unitdisk, paleyellow+opacity(0.25), nolight);
draw(scale3(2*sqrt(2))*unitcircle3, 0.8*red);

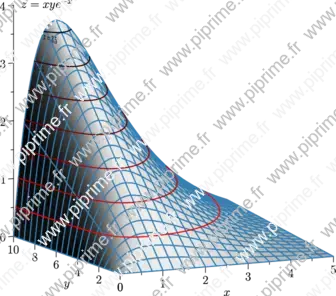
🔗graph3-fig009

Figure graph3 009 Generated with Asymptote

size(12cm,0,false);
import graph3;
import contour;
import palette;

texpreamble("\usepackage{icomma}");

real f(pair z) {return z.x*z.y*exp(-z.x);}

currentprojection=orthographic(-2.5,-5,1);

draw(surface(f,(0,0),(5,10),20,Spline),palegray,bp+rgb(0.2,0.5,0.7));

scale(true);

xaxis3(Label("$x$",MidPoint),OutTicks());
yaxis3(Label("$y$",MidPoint),OutTicks(Step=2));
zaxis3(Label("$z=xye^{-x}$",Relative(1),align=2E),Bounds(Min,Max),OutTicks);

real[] datumz={0.5,1,1.5,2,2.5,3,3.5};

Label[] L=sequence(new Label(int i) {
    return YZ()*(Label(format("$z=%g$",datumz[i]),
                       align=2currentprojection.vector()-1.5Z,Relative(1)));
  },datumz.length);

pen fontsize=bp+fontsize(10);
draw(L,lift(f,contour(f,(0,0),(5,10),datumz)),
     palette(datumz,Gradient(fontsize+red,fontsize+black)));

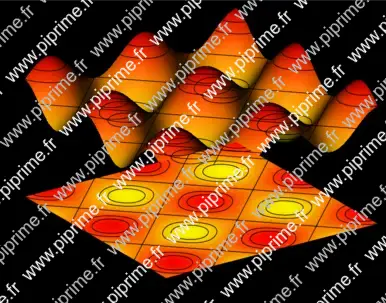
🔗graph3-fig011

Figure graph3 011 Generated with Asymptote

import graph3;
import palette;
import contour;
size(14cm,0);
currentprojection=orthographic(-1,-1.5,0.75);
currentlight=(-1,0,5);

real a=1, b=1;
real f(pair z) { return a*(6+sin(z.x/b)+sin(z.y/b));}
real g(pair z){return f(z)-6a;}

// The axes
limits((0,0,4a),(14,14,8a));
xaxis3(Label("$x$",MidPoint),OutTicks());
yaxis3(Label("$y$",MidPoint),OutTicks(Step=2));
ticklabel relativelabel()
{
  return new string(real x) {return (string)(x-6a);};
}
zaxis3(Label("$z$",Relative(1),align=2E),Bounds(Min,Max),OutTicks(relativelabel()));

// The surface
surface s=surface(f,(0,0),(14,14),100,Spline);

pen[] pens=mean(palette(s.map(zpart),Gradient(yellow,red)));

// Draw the surface
draw(s,pens);
// Project the surface onto the XY plane.
draw(planeproject(unitsquare3)*s,pens,nolight);

// Draw contour for "datumz"
real[] datumz={-1.5, -1, 0, 1, 1.5};
guide[][] pl=contour(g,(0,0),(14,14),datumz);
for (int i=0; i < pl.length; ++i)
  for (int j=0; j < pl[i].length; ++j)
    draw(path3(pl[i][j]));

// Draw the contours on the surface
draw(lift(f,pl));

if(!is3D())
  shipout(bbox(3mm,Fill(black)));