PIPRIME.FR BLOG

Live fully to honor life

Category Surveys -- Asymptote Gallery

đź”—ccw-fig001

Figure ccw 001 Generated with Asymptote

Usage of the algorithm "Simple Polygon Orientation".

Show ccw/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Path Orientation

size(8cm, false);

real signedArea(pair [] pt)
{
  // Return the signed area of a simple (NON CROSSED) polygon of vertex "pt"
  // Retourne l'aire algébrique d'un polygone NON CROISÉ
  pair [] pt_= copy(pt);
  real n=pt.length,
    sa=0;
  pt_.push(pt_[0]);
  pt_.push(pt_[1]);

  for (int i=1; i<=n; ++i) sa +=pt_[i].x * (pt_[i+1].y - pt_[i-1].y);
  return sa/2;
}

bool counterclockwise(pair [] pt)
{
  // Return "true" if the polygon (SIMPLE CURVE i.e. NON CROSSED)
  // of vertex "pt" is counterclockwise
  // Retourne "true" si le polygone (NON CROISÉ) de sommets "pt"
  // est dans le sens des aiguilles d'une montre
  return (signedArea(pt) > 0);
}

pair [] reverse(pair [] pt)
{
  pair [] pt_=copy(pt);
  int begin=0, end=pt.length-1;
  while (begin<end)
    {
      pair temp=pt_[begin];
      pt_[begin]=pt_[end];
      pt_[end]=temp;
      ++begin; --end;
    }
  return pt_;
}

pair [] counterclockdirected(pair [] pt)
{
  if (counterclockwise(pt)) return pt; else return reverse(pt);
}

path polygon(pair [] pt)
{
  int l=pt.length;
  guide opath;
  for (int i=0; i<l; ++i)
    {
      opath = opath--pt[i];
    }
  return opath;
}

pair [] pg = {(0,0), (1,0), (1,1), (2,2), (-1,1)};
draw(polygon(pg)--cycle,
     Arrow(Relative(.1)), BeginBar);
draw(polygon(counterclockdirected(reverse(pg)))--cycle,
     Arrow(position=Relative(.2), FillDraw(red)), BeginBar);

đź”—ccw-fig002

Figure ccw 002 Generated with Asymptote

Show ccw/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Path Orientation

size(6cm);
bool counterclockwise(path g, int n=10^3)
{
  // Return "true" if "g" (SIMPLE CURVE i.e. NON CROSSED) is counterclockwise
  // Retounre "true" si "g" (NON CROISÉ) est dans le sens contraire des aiguilles d'une montre
  if (!cyclic(g) || length(g)==0) abort("The function 'clocksize' needs cyclic path.");
  pair [] pt;
  real l=length(g),
    step=1/n,
    t=0,
    sa=0;
  do {
    sa +=point(g,t).x * (point(g,t+step).y - point(g,t-step).y);
    t+=step;
  } while (t<l);
  return (sgn(sa) > 0);
}

path counterclockdirected(path g,int n=10^3)
{
  // Return "g" (SIMPLE CURVE i.e. NON CROSSED) counterclockwise
  // Retourne "g" (NON CROISÉ) dans le sens des aiguilles d'une montre
  if (counterclockwise(g,n)) return g; else return reverse(g);
}

path p=unitcircle;
draw(counterclockdirected(reverse(p)),
     Arrow(Relative(.1)), BeginBar);
draw(counterclockdirected(p),
     Arrow(position=Relative(.2),FillDraw(red)), BeginBar);

đź”—ccw-fig003

Figure ccw 003 Generated with Asymptote

Use of the native windingnumber Asymptote routine this is the most fast and robust.

Utilisation du nombre d'enroulement avec la routine native windingnumber d'Asymptote c'est plus rapide et plus robuste.

Explanations are here.

Show ccw/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Path Orientation

size(6cm,0, false);
bool counterclockwise(path g, pair z) {return windingnumber(g,z) > 0;}

path counterclockdirected(path g,pair z)
{
  if (counterclockwise(g,z)) return g; else return reverse(g);
}

pair z=(1,0);
dot(z);
path p=(0,0){N}..(4,0){N}..cycle;
draw(counterclockdirected(reverse(p),z),Arrow(Relative(.1)), BeginBar);
draw(counterclockdirected(p,z),Arrow(position=Relative(.2),FillDraw(red)), BeginBar);

pair z=(3,-2);
dot(z);
path p=(4,-2){N}..(0,-2){N}..cycle;
draw(counterclockdirected(reverse(p),z),Arrow(Relative(.1)), BeginBar);
draw(counterclockdirected(p,z),Arrow(position=Relative(.2),FillDraw(red)), BeginBar);

pair z=(1,-4.5);
dot(z);
path p=yscale(.75)*((0,-6){N}..(2,-6){S}..(0,-6){N}..(4,-6){S}..cycle);
draw(counterclockdirected(reverse(p),z),Arrow(Relative(.1)), BeginBar);
draw(counterclockdirected(p,z),Arrow(position=Relative(.2),FillDraw(red)), BeginBar);

pair z=(3,-8);
dot(z);
path p=shift((0,-3.5))*p;
draw(counterclockdirected(reverse(p),z),Arrow(Relative(.1)), BeginBar);
draw(counterclockdirected(p,z),Arrow(position=Relative(.2),FillDraw(red)), BeginBar);

đź”—ccw-fig004

Figure ccw 004 Generated with Asymptote

Show ccw/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Path Orientation

// Use of the windingnumber works also for CROSSED curves

size(8cm,10cm,false);

import math;

bool counterclockwise(path g)
{
  // Return "true" if "g" is counterclockwise
  // Retounre "true" si "g" est dans le sens contraire des aiguilles d'une montre
  return (windingnumber(g,inside(g)) > 0);
}

path counterclockdirected(path g)
{
  if (counterclockwise(g)) return g; else return reverse(g);
}

guide p=randompath(30)..cycle;
draw(counterclockdirected(reverse(p)),Arrow(10bp,Relative(0.025)), BeginBar);
draw(counterclockdirected(p),Arrow(10bp,FillDraw(red),Relative(.05)), BeginBar);

đź”—fractales-fig001

Figure fractales 001 Generated with Asymptote

Show fractales/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Function (creating) | #Fractals | #Recursion | #Transform | #Picture

// From documentation of Asymptote
size(250);

real a=3;
real b=4;
real c=hypot(a,b);

transform ta=shift(c,c)*rotate(-aCos(a/c))*scale(a/c)*shift(-c);
transform tb=shift(0,c)*rotate(aCos(b/c))*scale(b/c);

picture Pythagorean(int n) {
  picture pic;
  fill(pic,scale(c)*unitsquare,1/(n+1)*green+n/(n+1)*brown);
  if(n == 0) return pic;
  picture branch=Pythagorean(--n);
  add(pic,ta*branch);
  add(pic,tb*branch);
  return pic;
}

add(Pythagorean(12));

đź”—fractales-fig002

Figure fractales 002 Generated with Asymptote

Show fractales/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Transform | #Function (creating)

size(10cm,0);

transform scale(pair center, real k) {
  return shift(center)*scale(k)*shift(-center);
}

path trk=(0,0)--(0,1);

void tree(path p, int n, real a=30, real b=40, real r=.75) {
  if (n!=0) {
    pair h=point(p,length(p));
    transform tb=rotate(180-b,h)*scale(h,r);
    transform ta=rotate(-180+a,h)*scale(h,r);
    draw(p,n/3+1/(n+1)*green+n/(n+1)*brown);
    tree(tb*reverse(p),n-1,a,b,r);
    tree(ta*reverse(p),n-1,a,b,r);
  }
}

tree(trk,12,a=25,b=40,r=.75);

đź”—fractales-fig003

Figure fractales 003 Generated with Asymptote

Show fractales/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Transform | #Picture | #Function (creating)

// Barnsley's fern
// Fougère de Barnsley

size(5cm,0);

real ab=85, ac=-5;
real rc=.85, rb=-.31;
path trk=(0,0)--(0,1);

transform ta=shift(0,1)*rotate(ab)*scale(rb);
transform tb=shift(0,1)*rotate(-ab)*scale(rb);
transform tc=shift(0,1)*rotate(ac)*scale(rc);

picture fern(int n) {
  picture opic;
  draw(opic,trk^^ta*trk^^tb*trk^^tc*trk);
  if (n==0) return opic;
  picture branch=fern(n-1);
  add(opic,branch);
  add(opic,ta*branch);
  add(opic,tb*branch);
  add(opic,tc*branch);
  return opic;
}

add(fern(6));

đź”—fractales-fig004

Figure fractales 004 Generated with Asymptote

Show fractales/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Transform | #Picture

// Barnsley's fern
// Fougère de Barnsley
size(10cm,0);

real ab=72, ac=-7;
real rc=0.85, rb=0.35;
path trk=(0,0)--(0,1);

transform ta=shift(0,1)*rotate(ab)*scale(rb);
transform tb=shift(0,1)*rotate(-ab)*scale(rb);
transform tc=shift(0,1)*rotate(ac)*scale(rc);
transform td=shift(0,1)*rotate((ab+ac)/2)*scale(rb);
transform te=shift(0,1)*rotate(-(ab+ac)/2)*scale(rb);

picture pic;
draw(pic,trk,red+.8green);

//Construct a fern branch as atractor
int nbit=7;
for(int i=1; i<=nbit; ++i) {
  picture pict;
  add(pict,ta*pic);
  add(pict,tb*pic);
  add(pict,tc*pic);
  draw(pict,(0,0)--(0,1), (2*(i/nbit)^2)*bp+((1-i/nbit)*green+i/nbit*brown));
  pic=pict;
}

//Use the fern branch to construct... a fern branch
picture pict;
add(pict,ta*pic);
add(pict,tb*pic);

pair x=(0,1);
nbit=23;
for(int i=1; i<=nbit; ++i) {
  add(shift(x)*rotate(ac*i)*scale(rc^i)*pict);
  draw(tc^i*((0,0)--(0,1)), 2*(1.5-i/nbit)^2*bp+brown);
  x=tc*x;
}

shipout(bbox(3mm, 2mm+black, FillDraw(paleyellow)));

đź”—fractales-fig005

Figure fractales 005 Generated with Asymptote

Show fractales/fig0050.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Transform | #Picture

// Barnsley's fern
// Fougère de Barnsley
size(5cm,0);

real ab=85, ac=-5;
real rc=0.8, rb=0.3;
path trk=(0,0)--(0,1);

transform [] t;
t[1] =shift(0,1)*rotate(ab)*scale(rb);
t[2] =shift(0,1)*rotate(-ab)*scale(rb);
t[3] =shift(0,1)*rotate(ac)*scale(rc);
real sum=0;

for(int i=0; i<100; ++i) sum+=(rc*cos(ac*pi/180))^i;
t[4] =xscale(0.01)*yscale(1/sum);

picture pic;
draw(pic,trk);
pair pt=(0,0);

for(int i=0; i < 1000; ++i) {
  pt=t[ 1+floor((3.0*rand()/randMax)) ]*pt;
}

int nbt;
for(int i=0; i < 200000; ++i) {
  nbt=1+floor((4.0*rand()/randMax));
  pt=t[nbt]*pt;
  draw(pt);
}

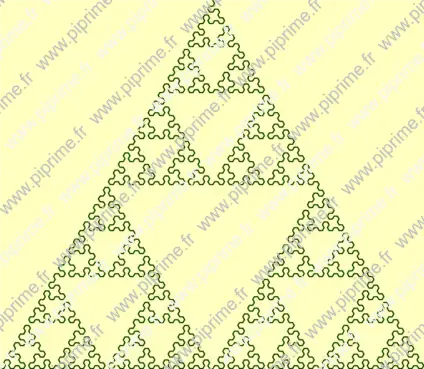
đź”—fractales-fig006

Figure fractales 006 Generated with Asymptote

Show fractales/fig0060.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Random | #Function (creating)

//From documentation of Asymptote
size(10cm);

// Draw Sierpinski triangle with top vertex A, side s, and depth q.
void Sierpinski(pair A, real s, int q,
                bool top=true, bool randcolor=false) {
  pair B=A-(1,sqrt(2))*s/2;
  pair C=B+s;
  if(top) draw(A--B--C--cycle);
  if (randcolor) {
    filldraw((A+B)/2--(B+C)/2--(A+C)/2--cycle,
             (.33*rand()/randMax*red+.33*rand()/randMax*green+.33*rand()/randMax*blue));
  } else draw((A+B)/2--(B+C)/2--(A+C)/2--cycle);
  if(q > 0) {
    Sierpinski(A,s/2,q-1,false,randcolor);
    Sierpinski((A+B)/2,s/2,q-1,false,randcolor);
    Sierpinski((A+C)/2,s/2,q-1,false,randcolor);
  }
}

Sierpinski((0,1), 1, 5, randcolor=true);

đź”—fractales-fig007

Figure fractales 007 Generated with Asymptote

Translate from http://zoonek.free.fr/LaTeX/Metapost/metapost.html

Show fractales/fig0070.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion

size(8cm);
void koch(pair A, pair B, int n) {
  pair C;
  C =rotate(120, point(A--B,1/3))*A;
  if (n>0) {
    koch( A,        point(A--B,1/3), n-1);
    koch( point(A--B,1/3), C,        n-1);
    koch( C,        point(A--B,2/3), n-1);
    koch( point(A--B,2/3), B,        n-1);
  } else draw(A--point(A--B,1/3)--C--point(A--B,2/3)--B);
}

pair z0=(1,0);
pair z1=rotate(120)*z0;
pair z2=rotate(120)*z1;
koch( z0, z1, 3 );
koch( z1, z2, 3 );
koch( z2, z0, 3 );

đź”—fractales-fig008

Figure fractales 008 Generated with Asymptote

Show fractales/fig0080.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Loop/for/while | #Picture

size(10cm,0);

real mandelbrot(pair c, real r, int count=100) {
  int i=0;
  pair z=c;
  do {
    ++i;
    z=z^2+c;
  } while (length(z) <= r && i<count);

  return (i<count) ? i/count : 0;
}

real r=4;
real step=.01;
real xmin=-2.25, xmax=.75;
real ymin=-1.3, ymax=0;

real x=xmin, y=ymin;
int xloop=round((xmax-xmin)/step);
int yloop=round((ymax-ymin)/step);
pen p;
path sq=scale(step)*unitsquare;

for(int i=0; i < xloop; ++i) {
  for(int j=0; j < yloop; ++j) {
    p=mandelbrot((x,y),r,20)*red;
    filldraw(shift(x,y)*sq,p,p);
    y += step;
  }
  x += step;
  y=ymin;
}

add(reflect((0,0),(1,0))*currentpicture);

đź”—fractales-fig009

Figure fractales 009 Generated with Asymptote

Show fractales/fig0090.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Transform | #Picture | #Array

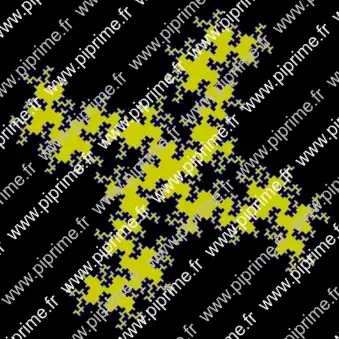
size(10cm,0);

real a=-1.5, b=2a/3;

picture H(pen p=currentpen) {
  picture H;
  draw(H,(-a,0)--(a,0)^^(-a,-b)--(-a,b)^^(a,-b)--(a,b),p);
  return H;
}

transform sc=scale(0.5);
transform[] t={identity(),
               shift(-a,b)*sc, shift(-a,-b)*sc,
               shift(a,b)*sc,  shift(a,-b)*sc};

picture Hfractal(int n, pen p=currentpen)
{
  picture pic;
  if(n == 0) return H(p);
  picture Ht=Hfractal(n-1,p);
  for (int i=0; i < 5; ++i) add(pic,t[i]*Ht);
  return pic;
}

add(Hfractal(4, bp+0.5*red));

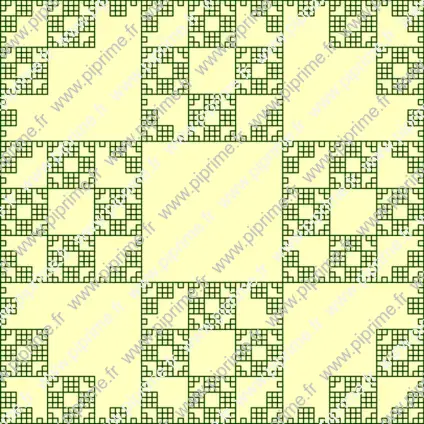
đź”—fractales-fig010

Figure fractales 010 Generated with Asymptote

Show fractales/fig0100.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Transform | #Picture | #Array

size(10cm,0);

real a=-1.5, b=2a/3;

path[] H=(-a,0)--(a,0)^^(-a,-b)--(-a,b)^^(a,-b)--(a,b);

transform sc=scale(0.5);
transform[] t={shift(-a,b)*sc, shift(-a,-b)*sc,
               shift(a,b)*sc,  shift(a,-b)*sc};

void Hfractal(path[] g, int n, pen[] p=new pen[]{currentpen})
{
  p.cyclic=true;
  if(n == 0) draw(H,p[0]); else {
    for (int i=0; i < 4; ++i) {
      draw(t[i]*g,p[n]);
      Hfractal(t[i]*g,n-1,p);
    }
  }
}

Hfractal(H, 5, new pen[] {0.8*red, 0.8*green, 0.8*blue, black, blue+red});

đź”—fractales-fig011

Figure fractales 011 Generated with Asymptote

Show fractales/fig0110.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Geometry | #Triangle | #Array | #Loop/for/while

import geometry;
size(10cm,0);

triangle[] dissect(triangle T, int n)
{
  if(n <= 0) return new triangle[]{T};
  triangle[] OT;
  point M=midpoint(T.BC);
  triangle[] Tp=dissect(triangle(M,T.A,T.B),n-1);
  for(triangle t : Tp) OT.insert(0,t);
  triangle[] Tp=dissect(triangle(M,T.C,T.A),n-1);
  for(triangle t : Tp) OT.insert(0,t);
  return OT;
}

triangle T=rotate(45)*triangle((1,1),(0,0),(2,0));
triangle[] DT=dissect(T,9);
path g;
transform R=reflect(T.BC);

for(int i : DT.keys) {
  draw(DT[i],miterjoin+0.9*red);
  draw(R*DT[i],miterjoin+0.9*red);
  g=g--centroid(DT[i]);
}

draw(scale(sqrt(2))*unitsquare,bp+miterjoin+0.8*blue);
draw(g--reverse(R*g)--cycle,bp+miterjoin+yellow);

shipout(bbox(sqrt(2)*mm, Fill(black)));

đź”—fractales-fig012

Figure fractales 012 Generated with Asymptote

Show fractales/fig0120.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Fractals
Tags : #Fractals | #Recursion | #Geometry | #Triangle | #Array | #Loop/for/while

size(12cm,0);

import geometry;

triangle T=triangleAbc(90,Tan(30),1);

triangle[] reverse(triangle[] arr)
{
  triangle[] or;
  int l=arr.length;
  for(int i=0; i < l; ++i) {
    or.push(arr[l-i-1]);
  }
  return or;
}

triangle[] dissect(triangle T, int n, bool reverse=false)
{
  if(n <= 0) return new triangle[]{T};
  triangle[] OT;

  point M=curpoint(T.AB,T.b()*Tan(30));
  point H=projection(T.BC)*M;
  triangle[] OT1, OT2, OT3;
  OT.append(dissect(triangle(H,T.B,M),n-1,!reverse));
  OT.append(reverse((dissect(triangle(H,T.C,M),n-1,!reverse))));
  OT.append(dissect(triangle(T.A,T.C,M),n-1,!reverse));
  return OT;
}

triangle[] DT=dissect(T,5);
point O=centroid(DT[0]);
path g;
transform Ro=rotate(30,T.B), Re=reflect(T.BC), Roj;

for(int i : DT.keys) {
  O=incenter(DT[i]);
  g=g--O;
}

g=reverse(g);
path G, g=g--Re*reverse(g) ;
for (int j=0; j < 12; j += 2) G=G--Ro^(-j)*g;

fill(G--cycle,0.3*blue);

for(int i : DT.keys) {
  for (int j=0; j < 12; j += 2) {
    Roj=Ro^j;
    draw(Roj*DT[i],miterjoin+0.8*red);
    draw(Roj*(Re*DT[i]),miterjoin+0.8*red);
  }
}

draw(G--cycle,bp+miterjoin+0.9*yellow);

shipout(bbox(2mm, FillDraw(black, 1mm+miterjoin+deepblue)));

đź”—lsystem-fig001

Figure lsystem 001 Generated with Asymptote

Show lsystem/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

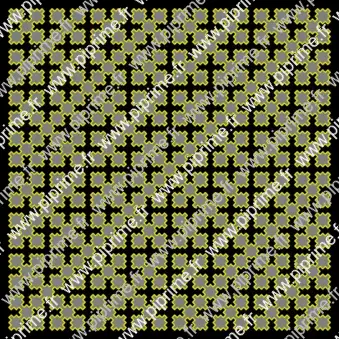
import Lsystem;
size(10cm);

string[][] rules={{"F","FF"}, {"X","--FXF++FXF++FXF--"}};
Lsystem Sierpinski=Lsystem("FXF--FF--FF", rules, La=60, Lai=0);
Sierpinski.iterate(5);
draw(Sierpinski.paths(), bp+0.2*green);

shipout(bbox(Fill(paleyellow)));

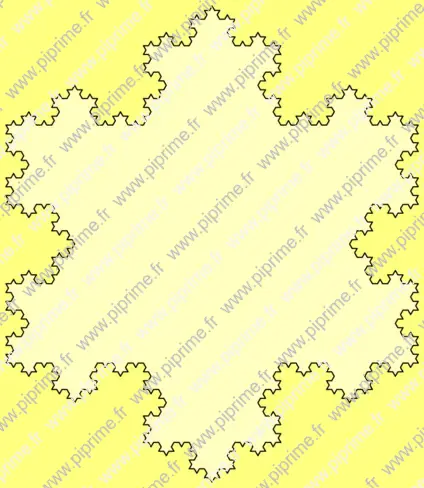
đź”—lsystem-fig002

Figure lsystem 002 Generated with Asymptote

Show lsystem/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(15cm,0);

Lsystem SierpinskiCurve=
  Lsystem("YF",
          new string[][]{{"X","YF+XF+Y"},{"Y","XF-YF-X"}},
          La=60, Lai=0);
SierpinskiCurve.iterate(7);
draw(SierpinskiCurve.paths(), bp+0.2*green);

shipout(bbox(Fill(paleyellow)));

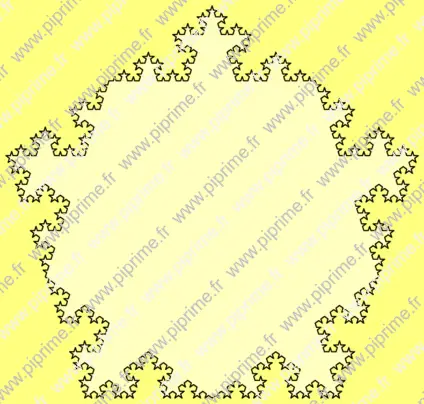
đź”—lsystem-fig003

Figure lsystem 003 Generated with Asymptote

Show lsystem/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(15cm,0);

Lsystem SierpinskiCarpet=
  Lsystem("F+F+F+F",
          new string[][]{{"F","FF+F+F+F+FF"}},
          La=90, Lai=0);
SierpinskiCarpet.iterate(4);
draw(SierpinskiCarpet.paths(), bp+0.2*green);

shipout(bbox(Fill(paleyellow)));

đź”—lsystem-fig004

Figure lsystem 004 Generated with Asymptote

Source

Show lsystem/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;

size(15cm,0);
Lsystem Koch=Lsystem("+F--F--F",
                     new string[][]{{"F","F+F--F+F"}},
                     La=60, Lai=0);
Koch.iterate(4);
filldraw(Koch.paths()[0]&cycle, paleyellow, 1bp+black);
shipout(bbox(2mm, Fill(lightyellow)));

đź”—lsystem-fig005

Figure lsystem 005 Generated with Asymptote

Show lsystem/fig0050.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;

size(15cm,0);
Lsystem Koch=Lsystem("+F+F+F+F+F",
                     new string[][]{{"F","F-F++F-F"}},
                     La=72);
Koch.iterate(4);
filldraw(Koch.paths()[0]&cycle, paleyellow, 1bp+black);
shipout(bbox(2mm, Fill(lightyellow)));

đź”—lsystem-fig006

Figure lsystem 006 Generated with Asymptote

Show lsystem/fig0060.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;

size(10cm,0);
Lsystem Koch=Lsystem("-F--F--F",
                     new string[][]{{"F","F-F++F-F"}},
                     La=60);
Koch.iterate(4);
filldraw(Koch.paths()[0]&cycle, paleyellow, 1bp+black);
shipout(bbox(2mm, Fill(lightyellow)));

đź”—lsystem-fig007

Figure lsystem 007 Generated with Asymptote

Show lsystem/fig0070.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

// Quadratic Koch Island
import Lsystem;
size(10cm,0);

string[][] rules={{"F","F-F+F+FFF-F-F+F"}};
Lsystem QuadraticKochIsland=Lsystem("F+F+F+F", rules, La=90, Lai=0);
QuadraticKochIsland.iterate(3);
draw(QuadraticKochIsland.paths(), bp+0.9*yellow);
shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig008

Figure lsystem 008 Generated with Asymptote

Source.

Show lsystem/fig0080.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,0);

Lsystem HilbertCurve=Lsystem("X",
                             new string[][]{{"X","-YF+XFX+FY-"},{"Y","+XF-YFY-FX+"}},
                             La=90, Lai=0);
HilbertCurve.iterate(6);
draw(HilbertCurve.paths(), linewidth(1bp));

shipout(bbox(Fill(lightgrey)));

đź”—lsystem-fig009

Figure lsystem 009 Generated with Asymptote

Show lsystem/fig0090.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,0);

Lsystem Fass=Lsystem("-L",
                     new string[][]{{"L","LFLF+RFR+FLFL-FRF-LFL-FR+F+RF-LFL-FRFRFR+"},
                                    {"R","-LFLFLF+RFR+FL-F-LF+RFR+FLF+RFRF-LFL-FRFR"}},
                     La=90);
Fass.iterate(3);
draw(Fass.paths(), linewidth(1bp));

shipout(bbox(Fill(lightgrey)));

đź”—lsystem-fig010

Figure lsystem 010 Generated with Asymptote

Show lsystem/fig0100.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,0);
currentprojection=currentprojection=orthographic((10,5,6));

string[][] rules={{"X","^<XF^<XFX-F^>>XFX&F+>>XFX-F>X->"}};
Lsystem3 HilbertCurve3D=Lsystem3("X", rules, La=90);
HilbertCurve3D.iterate(3);

// !! Use a lot of memory !!
/* path3[] g=HilbertCurve3D.paths3();
   draw(g[0], linewidth(bp)+0.9*yellow);
*/

HilbertCurve3D.drawpaths3(linewidth(bp)+0.9*yellow);

shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig011

Figure lsystem 011 Generated with Asymptote

Show lsystem/fig0110.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(15cm,0);

string[][] rules={{"F","FF"},
                  {"H","+F+FH++FFH++F+FF+FH++FFH++F+F-"}};

Lsystem H=Lsystem("+H",rules, La=90);
H.iterate(5);
draw(H.paths(), white);

shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig012

Figure lsystem 012 Generated with Asymptote

Source.

Show lsystem/fig0120.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,0);

string[][] rules=new string[][]{{"F",""},{"X","-FX++FY-"},{"Y","+FX--FY+"}};
Lsystem dragon=Lsystem("X",rules, La=45, Lai=0);
dragon.iterate(12);
draw(dragon.paths(), white);

shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig013

Figure lsystem 013 Generated with Asymptote

Show lsystem/fig0130.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(12cm,0);

string[][] rules={{"F","-F+F-F-F+F+FF-F+F+FF+F-F-FF+FF-FF+F+F-FF-F-F+FF-F-F+F+F-F+"}};
Lsystem segment32Curve= Lsystem("F+F+F+F", rules, La=90, Lai=45);
segment32Curve.iterate(2);
filldraw(segment32Curve.paths()[0]&cycle, 0.8*yellow, blue);
shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig014

Figure lsystem 014 Generated with Asymptote

Show lsystem/fig0140.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

// Peano Gosper curve
import Lsystem;
size(12cm,0);

Lsystem PeanoGosperCurve=
  Lsystem("FX",
          new string[][]{{"X","X+YF++YF-FX--FXFX-YF+"},{"Y","-FX+YFYF++YF+FX--FX-Y"}},
          La=60, Lai=0);
PeanoGosperCurve.iterate(4);
draw(PeanoGosperCurve.paths(), bp+0.9*yellow);

shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig015

Figure lsystem 015 Generated with Asymptote

Show lsystem/fig0150.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(12cm,0);

string[][] rules={{"X","XF-F+F-XF+F+XF-F+F-X"}};
Lsystem squareCurve= Lsystem("F+XF+F+XF", rules, La=90, Lai=45);
squareCurve.iterate(5);
filldraw(squareCurve.paths()[0]&cycle, grey, 1bp+0.9*yellow);
shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig016

Figure lsystem 016 Generated with Asymptote

Show lsystem/fig0160.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(12cm,0);

string[][] rules={{"L","+R-F-R+"}, {"R","-L+F+L-"}};
Lsystem squareCurve= Lsystem("L--F--L--F", rules, La=45, Lai=45);
squareCurve.iterate(9);
filldraw(squareCurve.paths()[0]&cycle, grey, 1bp+0.9*yellow);

shipout(bbox(3mm, Fill(black)));

đź”—lsystem-fig017

Figure lsystem 017 Generated with Asymptote

Show lsystem/fig0170.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,0);

string[][] rules={
  {"F", "F+f-FF+F+FF+Ff+FF-f+FF-F-FF-Ff-FFF"},
  {"f", "ffffff"}
};

Lsystem oer=Lsystem("F+F+F+F",rules,La=91);

oer.iterate(2);
draw(oer.paths(), bp+0.8*yellow);
shipout(bbox(2mm, Fill(black)));

đź”—lsystem-fig018

Figure lsystem 018 Generated with Asymptote

Show lsystem/fig0180.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(15cm,0);

string[][] rules={
  {"F", ""},
  {"P", "--FR++++FS--FU"},
  {"Q", "FT++FR----FS++"},
  {"R", "++FP----FQ++FT"},
  {"S", "FU--FP++++FQ--"},
  {"T", "+FU--FP+"},
  {"U", "-FQ++FT-"}
};

Lsystem pentive=Lsystem("Q", rules, La=36, Lai=180);

pentive.iterate(8);
draw(pentive.paths(), 0.8*yellow);
shipout(bbox(2mm, Fill(black)));

đź”—lsystem-fig019

Figure lsystem 019 Generated with Asymptote

Show lsystem/fig0190.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(12cm,0);

string[][] rules={
  {"A", "X+X+X+X+X+X+"},
  {"X", "[F+F+F+F[---X-Y]+++++F++++++++F-F-F-F]"},
  {"Y", "[F+F+F+F[---Y]+++++F++++++++F-F-F-F]"}
};

Lsystem spiral=Lsystem("AAAA",rules,La=15);

spiral.iterate(6);
draw(spiral.paths(), 0.9*green);

shipout(bbox(2mm, Fill(black)));

đź”—lsystem-fig020

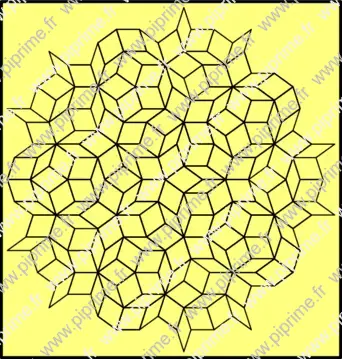
Figure lsystem 020 Generated with Asymptote

Rhombus tiling (P3)).

Show lsystem/fig0200.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Tiling

import Lsystem;
size(12cm,0);

string[][] rules={
  {"6","8F++9F----7F[-8F----6F]++"},
  {"7","+8F--9F[---6F--7F]+"},
  {"8","-6F++7F[+++8F++9F]-"},
  {"9","--8F++++6F[+9F++++7F]--7F"},
  {"F",""}
};

Lsystem Penrose=Lsystem("[7]++[7]++[7]++[7]++[7]", rules, La=36);
Penrose.iterate(4);
draw(Penrose.paths(), linewidth(bp));

shipout(bbox(2mm, FillDraw(lightyellow,linewidth(1mm))));

đź”—lsystem-fig021

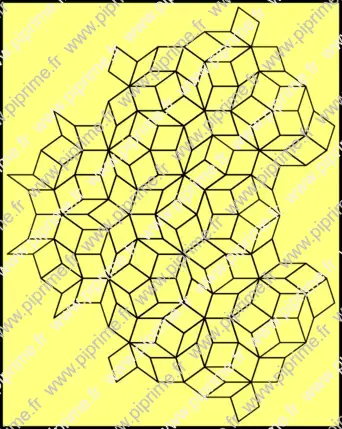
Figure lsystem 021 Generated with Asymptote

Penrose tiling

Show lsystem/fig0210.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Tiling

import Lsystem;
size(12cm,0);

string[][] rules={
  {"W", "YF++ZF----XF[-YF----WF]++"},
  {"X", "+YF--ZF[---WF--XF]+"},
  {"Y", "-WF++XF[+++YF++ZF]-"},
  {"Z", "--YF++++WF[+ZF++++XF]--XF"},
  {"F", ""}
};

Lsystem Penrose=Lsystem("++ZF----XF-YF----WF",rules,La=36);

Penrose.iterate(4);
draw(Penrose.paths(), linewidth(bp));

shipout(bbox(2mm, FillDraw(lightyellow,linewidth(1mm))));

đź”—lsystem-fig022

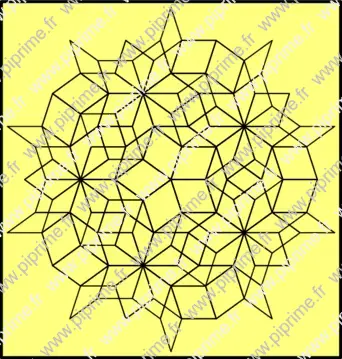
Figure lsystem 022 Generated with Asymptote

Show lsystem/fig0220.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Tiling

import Lsystem;
size(12cm,0);

string[][] rules={
  {"W", "YF++ZF----XF[-YF----WF]++"},
  {"X", "+YF--ZF[---WF--XF]+"},
  {"Y", "-WF++XF[+++YF++ZF]-"},
  {"Z", "--YF++++WF[+ZF++++XF]--XF"},
  {"F", ""}
};

Lsystem Penrose=Lsystem("[X][Y]++[X][Y]++[X][Y]++[X][Y]++[X][Y]",rules,La=36);

Penrose.iterate(3);
draw(Penrose.paths(), linewidth(bp));

shipout(bbox(2mm, FillDraw(lightyellow,linewidth(1mm))));

đź”—lsystem-fig023

Figure lsystem 023 Generated with Asymptote

Inspiration.

Show lsystem/fig0230.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

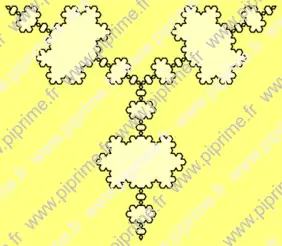
import Lsystem;
size(10cm, 10cm);
settings.outformat="pdf"; // for opacity

string[][] rules=new string[][]{{"F", "FF-[-F+F+F]+[+F-F-F]"}};
Lsystem plant=Lsystem("F", rules, La=22.5, Lai=90);
plant.iterate(5);
path[] g=plant.paths();

tree g=plant.tree();

for (int i:g.keys) {
  if((g[i].depth == 0)) draw(g[i].g, yellow);
}

for (int i:g.keys) {
  if((g[i].depth > 0 )) draw(g[i].g, green+opacity(0.5));
}

for (int i:g.keys) {
  if((g[i].depth > 15)) draw(g[i].g, brown+opacity(0.3));
}

shipout(bbox(Fill(paleyellow)));

đź”—lsystem-fig024

Figure lsystem 024 Generated with Asymptote

Inspirtaion.

Show lsystem/fig0240.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | L-System
Tags : #L-System | #Fractals

import Lsystem;
size(10cm,10cm);
settings.outformat="pdf"; // for opacity

string[][] rules=new string[][]{{"F", "FF"},{"X", "F-[[X]+X]+F[+FX]-X"}};
Lsystem plant=Lsystem("X", rules, La=22.5, Lai=90);
plant.iterate(8);
path[] g=plant.paths();

tree g=plant.tree();

for (int i:g.keys) {
  if((g[i].depth <= 2 )) draw(g[i].g, yellow);
}

for (int i:g.keys) {
  if((g[i].depth > 2 ) && (g[i].depth <= 10 )) draw(g[i].g, green+opacity(0.5));
}

for (int i:g.keys) {
  if((g[i].depth > 11)) draw(g[i].g, brown+opacity(0.3));
}

shipout(bbox(Fill(paleyellow)));

đź”—randomwalk-fig001

Figure randomwalk 001 Generated with Asymptote

Show randomwalk/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random | #Function (creating) | #Array | #Loop/for/while

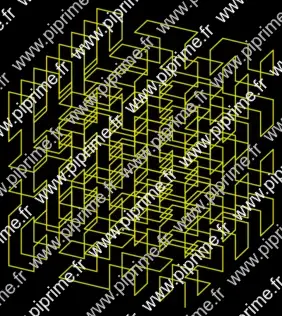
import three;
settings.render=0;

// The available directions of steps
triple[] dirs={X,-X,Y,-Y,Z,-Z};
dirs.cyclic=true;

// Return the nodes of the path
triple[] randWalk(real Srnd(), int n)
{
  triple[] randPath;
  triple camera=1e10*currentprojection.camera;
  triple pos=O, tpos;
  int R;
  for (int i=0; i < n; ++i) {
    R=round(Srnd());
    tpos=pos+dirs[R];
    randPath.push(tpos);
    pos=tpos;
  }
  return randPath;
}
triple[] randWalk(int Srnd(), int n)
{
  real R(){ return Srnd();}
  return randWalk(R,n);
}

void drawWalk(triple[] nodes, pen p=white)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(nodes)),abs(maxbound(nodes)));
  real[][] depth;
  for(int i=0; i < nodes.length-1; ++i) {
    real d=abs(camera-0.5*(nodes[i]+nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=nodes[round(depth[0][1])];
  triple m=nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(nodes[i]--nodes[i+1],abs(nodes[i]-m)/abs(M-m)*p);
  }
}


size(18cm);
currentprojection=orthographic((1,1,1));

drawWalk(randWalk(rand,50000),cyan);
shipout(bbox(3mm,Fill));

đź”—randomwalk-fig002

Figure randomwalk 002 Generated with Asymptote

Show randomwalk/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random | #Struct

import three;
settings.render=0;

// The available directions of steps
triple[] dirs={X,-X,Y,-Y,Z,-Z};
dirs.cyclic=true;

struct walk
{
  triple[] nodes;
  pen[] p;
}

// Comput the nodes of the path
walk randWalk(real Srnd(), int n, pen[] p={currentpen})
{
  p.cyclic=true;
  walk ow;
  triple pos=O, tpos;
  for (int i=0; i < n; ++i) {
    int R=round(Srnd());
    tpos=pos+dirs[R];
    ow.nodes.push(tpos);
    ow.p.push(p[R]);
    pos=tpos;
  }
  return ow;
}

walk randWalk(int Srnd(), int n, pen[] p={currentpen})
{
  real R(){ return Srnd();}
  return randWalk(R,n,p);
}

void drawWalk(walk walk)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(walk.nodes)),abs(maxbound(walk.nodes)));
  real[][] depth;
  for(int i=0; i < walk.nodes.length-1; ++i) {
    real d=abs(camera-0.5*(walk.nodes[i]+walk.nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=walk.nodes[round(depth[0][1])];
  triple m=walk.nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    // dot(walk.nodes[i],walk.p[i]);
    draw(walk.nodes[i]--walk.nodes[i+1],abs(walk.nodes[i]-m)/abs(M-m)*(walk.p[i]+walk.p[i+1]));
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(rand,50000,new pen[]{red, blue, green, yellow, purple}));
shipout(bbox(3mm,Fill));

đź”—randomwalk-fig003

Figure randomwalk 003 Generated with Asymptote

Show randomwalk/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random

import three;
import stats;
settings.render=0;

string[] dirs={"U","D","B","F","R","L"};
// U=up, D=down, B=backward, F=forward, R=right, L=left
dirs.cyclic=true;

// Comput the nodes of the path
triple[] randWalk(real Srnd(), int n, real angle=90)
{
  triple[] randPath;
  triple udir=Z, vdir=X, kdir=cross(udir,vdir);
  triple pos=O, tpos;
  void changedir(real angle, triple axe)
  {
    transform3 T=rotate(angle,axe);
    udir=T*udir;
    vdir=T*vdir;
    kdir=T*kdir;
  }
  void nextdir()
  {
    string R=dirs[round(Srnd())];
    if(R == "R") changedir(-angle,kdir);
    else if(R == "L") changedir(angle,kdir);
    else if(R == "U") changedir(angle,vdir);
    else if(R == "D") changedir(-angle,vdir);
    else if(R == "B") changedir(180,udir);
  }
  for (int i=0; i < n; ++i) {
    tpos=pos+udir;
    randPath.push(tpos);
    pos=tpos;
    nextdir();
  }
  return randPath;
}

triple[] randWalk(int Srnd(), int n, real angle=90)
{
  real R(){ return Srnd();}
  return randWalk(R,n,angle);
}

void drawWalk(triple[] nodes, pen p=white)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(nodes)),abs(maxbound(nodes)));
  real[][] depth;
  for(int i=0; i < nodes.length-1; ++i) {
    real d=abs(camera-0.5*(nodes[i]+nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=nodes[round(depth[0][1])];
  triple m=nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(nodes[i]--nodes[i+1],abs(nodes[i]-M)/abs(M-m)*p);
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(Gaussrand,50000));

đź”—randomwalk-fig004

Figure randomwalk 004 Generated with Asymptote

Show randomwalk/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random

import three;
import stats;
settings.render=0;

struct walk
{
  triple[] nodes;
  pen[] p;
}

string[] dirs={"U","D","B","F","R","L"};
// U=up, D=down, B=backward, F=forward, R=right, L=left
dirs.cyclic=true;

// Comput the nodes of the path
walk randWalk(real Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  p.cyclic=true;
  walk ow;
  triple udir=Z, vdir=X, kdir=cross(udir,vdir);
  triple pos=O, tpos;
  void changedir(real angle, triple axe)
  {
    transform3 T=rotate(angle,axe);
    udir=T*udir;
    vdir=T*vdir;
    kdir=T*kdir;
  }
  void nextdir()
  {
    int rd=round(Srnd());
    ow.p.push(p[rd]);
    string R=dirs[rd];
    if(R == "R") changedir(-angle,kdir);
    else if(R == "L") changedir(angle,kdir);
    else if(R == "U") changedir(angle,vdir);
    else if(R == "D") changedir(-angle,vdir);
    else if(R == "B") changedir(180,udir);
  }
  for (int i=0; i < n; ++i) {
    tpos=pos+udir;
    ow.nodes.push(tpos);
    pos=tpos;
    nextdir();
  }
  return ow;
}

walk randWalk(int Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  real R(){ return Srnd();}
  return randWalk(R,n,angle,p);
}

void drawWalk(walk walk)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(walk.nodes)),abs(maxbound(walk.nodes)));
  real[][] depth;
  for(int i=0; i < walk.nodes.length-1; ++i) {
    real d=abs(camera-0.5*(walk.nodes[i]+walk.nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=walk.nodes[round(depth[0][1])];
  triple m=walk.nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(walk.nodes[i]--walk.nodes[i+1],
         abs(walk.nodes[i]-m)/abs(M-m)*walk.p[i]);
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(Gaussrand,50000,new pen[] {red,yellow,blue}));
shipout(bbox(3mm,Fill));

đź”—randomwalk-fig005

Figure randomwalk 005 Generated with Asymptote

Show randomwalk/fig0050.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random

import three;
import stats;
settings.render=0;

struct walk
{
  triple[] nodes;
  pen[] p;
}

string[] dirs={"U","D","B","F","R","L"};
// U=up, D=down, B=backward, F=forward, R=right, L=left
dirs.cyclic=true;

// Comput the nodes of the path
walk randWalk(real Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  p.cyclic=true;
  walk ow;
  triple udir=Z, vdir=X, kdir=cross(udir,vdir);
  triple pos=O, tpos;
  void changedir(real angle, triple axe)
  {
    transform3 T=rotate(angle,axe);
    udir=T*udir;
    vdir=T*vdir;
    kdir=T*kdir;
  }
  void nextdir()
  {
    int rd=round(Srnd());
    ow.p.push(p[rd]);
    string R=dirs[rd];
    if(R == "R") changedir(-angle,kdir);
    else if(R == "L") changedir(angle,kdir);
    else if(R == "U") changedir(angle,vdir);
    else if(R == "D") changedir(-angle,vdir);
    else if(R == "B") changedir(180,udir);
  }
  for (int i=0; i < n; ++i) {
    tpos=pos+udir;
    ow.nodes.push(tpos);
    pos=tpos;
    nextdir();
  }
  return ow;
}

walk randWalk(int Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  real R(){ return Srnd();}
  return randWalk(R,n,angle,p);
}

void drawWalk(walk walk)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(walk.nodes)),abs(maxbound(walk.nodes)));
  real[][] depth;
  for(int i=0; i < walk.nodes.length-1; ++i) {
    real d=abs(camera-0.5*(walk.nodes[i]+walk.nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=walk.nodes[round(depth[0][1])];
  triple m=walk.nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(walk.nodes[i]--walk.nodes[i+1],
         abs(walk.nodes[i]-m)/abs(M-m)*walk.p[i]);
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(Gaussrand,1000,60,new pen[] {red,yellow,blue}));
shipout(bbox(3mm,Fill));

đź”—randomwalk-fig006

Figure randomwalk 006 Generated with Asymptote

Show randomwalk/fig0060.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random

import three;
import stats;
settings.render=0;

struct walk
{
  triple[] nodes;
  pen[] p;
}

string[] dirs={"U","D","B","F","R","L"};
// U=up, D=down, B=backward, F=forward, R=right, L=left
dirs.cyclic=true;

// Comput the nodes of the path
walk randWalk(real Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  p.cyclic=true;
  walk ow;
  triple udir=Z, vdir=X, kdir=cross(udir,vdir);
  triple pos=O, tpos;
  void changedir(real angle, triple axe)
  {
    transform3 T=rotate(angle,axe);
    udir=T*udir;
    vdir=T*vdir;
    kdir=T*kdir;
  }
  void nextdir()
  {
    int rd=round(Srnd());
    ow.p.push(p[rd]);
    string R=dirs[rd];
    if(R == "R") changedir(-angle,kdir);
    else if(R == "L") changedir(angle,kdir);
    else if(R == "U") changedir(angle,vdir);
    else if(R == "D") changedir(-angle,vdir);
    else if(R == "B") changedir(180,udir);
  }
  for (int i=0; i < n; ++i) {
    tpos=pos+udir;
    ow.nodes.push(tpos);
    pos=tpos;
    nextdir();
  }
  return ow;
}

walk randWalk(int Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  real R(){ return Srnd();}
  return randWalk(R,n,angle,p);
}

void drawWalk(walk walk)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(walk.nodes)),abs(maxbound(walk.nodes)));
  real[][] depth;
  for(int i=0; i < walk.nodes.length-1; ++i) {
    real d=abs(camera-0.5*(walk.nodes[i]+walk.nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=walk.nodes[round(depth[0][1])];
  triple m=walk.nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(walk.nodes[i]--walk.nodes[i+1],
         abs(walk.nodes[i]-m)/abs(M-m)*walk.p[i]);
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(Gaussrand,10000,60,new pen[] {red,yellow,blue}));
shipout(bbox(3mm,Fill));

đź”—randomwalk-fig007

Figure randomwalk 007 Generated with Asymptote

Show randomwalk/fig0070.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Random Walk (3D)
Tags : #Random

import three;
settings.render=0;

struct walk
{
  triple[] nodes;
  pen[] p;
}

string[] dirs={"U","D","B","F","R","L"};
// U=up, D=down, F=forward, R=right, L=left
dirs.cyclic=true;

// Comput the nodes of the path
walk randWalk(real Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  p.cyclic=true;
  walk ow;
  triple udir=Z, vdir=X, kdir=cross(udir,vdir);
  triple pos=O, tpos;
  void changedir(real angle, triple axe)
  {
    transform3 T=rotate(angle,axe);
    udir=T*udir;
    vdir=T*vdir;
    kdir=T*kdir;
  }
  void nextdir()
  {
    int rd=round(Srnd());
    ow.p.push(p[rd]);
    string R=dirs[rd];
    if(R == "R") changedir(-angle,kdir);
    else if(R == "L") changedir(angle,kdir);
    else if(R == "U") changedir(angle,vdir);
    else if(R == "D") changedir(-angle,vdir);
    else if(R == "B") changedir(180,udir);
  }
  for (int i=0; i < n; ++i) {
    tpos=pos+udir;
    ow.nodes.push(tpos);
    pos=tpos;
    nextdir();
  }
  return ow;
}

walk randWalk(int Srnd(), int n, real angle=90, pen[] p={currentpen})
{
  real R(){ return Srnd();}
  return randWalk(R,n,angle,p);
}

void drawWalk(walk walk)
{
  triple camera=currentprojection.camera;
  if(currentprojection.infinity)
    camera *= max(abs(minbound(walk.nodes)),abs(maxbound(walk.nodes)));
  real[][] depth;
  for(int i=0; i < walk.nodes.length-1; ++i) {
    real d=abs(camera-0.5*(walk.nodes[i]+walk.nodes[i+1]));
    depth.push(new real[] {d,i});
  }
  depth=sort(depth);
  triple M=walk.nodes[round(depth[0][1])];
  triple m=walk.nodes[round(depth[depth.length-1][1]+1)];
  // Draw from farthest to nearest
  while(depth.length > 0) {
    real[] a=depth.pop();
    int i=round(a[1]);
    draw(walk.nodes[i]--walk.nodes[i+1],
         abs(walk.nodes[i]-m)/abs(M-m)*walk.p[i]);
  }
}


size(18cm);
currentprojection=orthographic((0.5,0.5,1));

drawWalk(randWalk(rand,10000,60,new pen[] {red,yellow,blue}));
shipout(bbox(3mm,Fill));

đź”—tiling-fig001

Figure tiling 001 Generated with Asymptote

Show tiling/fig0010.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling | #Function (creating) | #Picture

size(10cm,0);

picture pavehexagonal(int depth=1)
{
  picture opic;
  path hexa=polygon(6);
  pair center;
  real a,ap,r,rp,r_d=180/pi;

  for(int j=0; j<depth; ++j)
    {
      for (int i=1; i<=6; ++i)
	{
	  a=i*60-30;
	  r=j*sqrt(3);
	  center=r*(rotate(a)*(1,0));
	  filldraw(opic, shift(center)*hexa, j/depth*.8red+(1-j/depth)*.8*blue);
	  //Uncomment to see centers of hexagons
	  dot(opic, shift(center)*midpoint(point(hexa,0)--point(hexa,3)));
	  //Uncomment to see circles passing by centers
	  //draw(opic, scale(r)*unitcircle, j/depth*red+(1-j/depth)*blue);
	  rp=r;
	  ap=0;
	  for (real k=0; k<j-1; ++k)
	    {
	      r=sqrt((1.5*(j-1 - k))^2 + 3/4*(j+1 + k)^2);
	      ap+=r_d*acos((rp^2 + r^2 - 3)/(2*r*rp));
	      center=r*(rotate(a + ap)*(1,0));
	      filldraw(opic, shift(center)*hexa, j/depth*.8*red+(1-j/depth)*.8*blue);
	      //Uncomment to see the centers of hexagons
	      //dot(opic, shift(center)*midpoint(point(hexa,0)--point(hexa,3)));
	      rp=r;
	      //Uncomment to see circles passing by centers
	      //draw(opic, scale(r)*unitcircle, j/depth*red+(1-j/depth)*blue);
            }
        }
    }
  return opic;
}


add(pavehexagonal(7));

đź”—tiling-fig002

Figure tiling 002 Generated with Asymptote

Show tiling/fig0020.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling | #Clip

size(6cm,0);

//Circular paving with the unit hexagonal picture "hexa"
picture pavehexagonal(picture hexa, int depth=1)
{
  picture opic;
  pair center;
  real a,ap,r,rp,r_d=180/pi;

  add(opic, hexa);

  for(int j=0; j<depth; ++j)
    {
      for (int i=1; i<=6; ++i)
	{
	  a=i*60-30;
	  r=j*sqrt(3);
	  center=r*(rotate(a)*(1,0));
	  add(opic, shift(center)*hexa);
	  rp=r;
	  ap=0;
	  for (real k=0; k<j-1; ++k)
	    {
	      r=sqrt((1.5*(j-1 - k))^2 + 3/4*(j+1 + k)^2);
	      ap+=r_d*acos((rp^2 + r^2 - 3)/(2*r*rp));
	      center=r*(rotate(a + ap)*(1,0));
	      add(opic, shift(center)*hexa);
	      rp=r;
	    }
	}
    }
  return opic;
}

picture hexa;
fill(hexa, polygon(6));
path inh=(0,0)--(.6,sqrt(3)/4)--(.5,sqrt(3)/2)--cycle;

for(int i=0; i<6; ++i)
  {
    fill(hexa, rotate(60*i)*inh,.5red);
  }

draw(hexa, inh);
add(rotate(45)*pavehexagonal(hexa,10));
clip(scale(10)*shift(-.5,-.5)*unitsquare);

đź”—tiling-fig003

Figure tiling 003 Generated with Asymptote

Show tiling/fig0030.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Path | #Picture | #Tiling

size(10cm,0);

transform r60=rotate(60);

pair A=(sqrt(3)/2,-.5);
pair B=r60*A, C=r60*B, D=r60*C, E=r60*D, F=r60*E;

path AB=A{dir(90)}..(.6,.5)..B{dir(0)};
path DE=shift(E-A)*reverse(AB);
path BC=B{dir(45)}..(.75,.7){dir(150)}..{dir(135)}(.65,.75){dir(70)}..(.5,1.25)..C{dir(255)};
path EF=shift(F-B)*reverse(BC);
path CD=C{dir(255)}..(-.4,.5){dir(200)}..D{dir(160)};
path FA=shift(A-C)*reverse(CD);

draw(A--B--C--D--E--F--cycle,linewidth(2pt));
draw(AB,2pt+.8red);
draw(DE,2pt+.8red);
draw(BC,2pt+.8blue);
draw(EF,2pt+.8blue);
draw(CD,2pt+.8green);
draw(FA,2pt+.8green);

picture hexa;
picture eye;

filldraw(hexa,AB--BC--CD--DE--EF--FA--cycle,black,white);
filldraw(eye,rotate(5)*xscale(.4)*unitcircle,white);
filldraw(hexa,subpath(AB,1,2)--subpath(BC,0,2){dir(225)}..{dir(245)}cycle,.1red+yellow,white);
draw(hexa,point(BC,0.1){dir(115)}.. (.8,.55) ..(.6,.65){dir(180)},yellow+grey);
filldraw(eye,rotate(5)*xscale(.4)*unitcircle,white);
fill(eye,rotate(5)*shift(0,-.1)*xscale(.25)*scale(.5)*unitcircle);
add(hexa,shift(.6,.9)*scale(.1)*eye);

add(shift(3,0)*hexa);

đź”—tiling-fig004

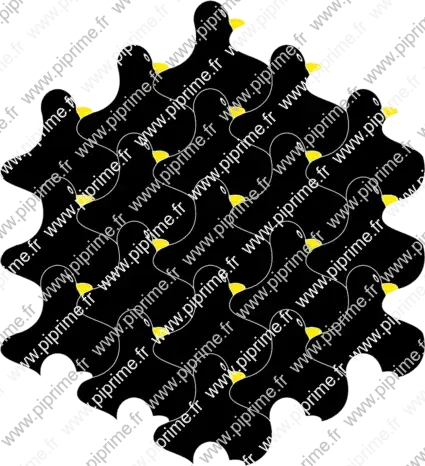
Figure tiling 004 Generated with Asymptote

Show tiling/fig0040.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling

size(15cm,0);

transform r60=rotate(60);
picture hexa;
picture eye;

pair A=(sqrt(3)/2,-.5);
pair B=r60*A, C=r60*B, D=r60*C, E=r60*D, F=r60*E;

//Body - corps
path AB=A{dir(90)}..(.6,.5)..B{dir(0)};
path DE=shift(E-A)*reverse(AB);
path BC=B{dir(45)}..(.75,.7){dir(150)}..{dir(135)}(.65,.75){dir(70)}..(.5,1.25)..C{dir(255)};
path EF=shift(F-B)*reverse(BC);
path CD=C{dir(255)}..(-.4,.5){dir(200)}..D{dir(160)};
path FA=shift(A-C)*reverse(CD);

filldraw(hexa,AB--BC--CD--DE--EF--FA--cycle,black,white);

//Nozzle - bec
filldraw(hexa,subpath(AB,1,2)--subpath(BC,0,2){dir(225)}..{dir(245)}cycle,.1red+yellow,white);
draw(hexa,point(BC,0.1){dir(115)}.. (.8,.55) ..(.6,.65){dir(180)},yellow+grey);

//Eye - oeil
filldraw(eye,rotate(5)*xscale(.4)*unitcircle,white);
filldraw(eye,rotate(5)*xscale(.4)*unitcircle,white);
fill(eye,rotate(5)*shift(0,-.1)*xscale(.25)*scale(.5)*unitcircle);
add(hexa,shift(.6,.9)*scale(.1)*eye);

//Circular paving with the unit hexagonal picture "hexa"
picture pavehexagonal(picture hexa, int depth=1)
{
  picture opic;
  pair center;
  real a,ap,r,rp,r_d=180/pi;

  add(opic, hexa);

  for(int j=0; j<depth; ++j)
    {
      for (int i=1; i<=6; ++i)
 {
   a=i*60-30;
   r=j*sqrt(3);
   center=r*(rotate(a)*(1,0));
   add(opic, shift(center)*hexa);
   rp=r;
   ap=0;
   for (real k=0; k<j-1; ++k)
     {
       r=sqrt((1.5*(j-1 - k))^2 + 3/4*(j+1 + k)^2);
       ap+=r_d*acos((rp^2 + r^2 - 3)/(2*r*rp));
       center=r*(rotate(a + ap)*(1,0));
       add(opic, shift(center)*hexa);
       rp=r;
     }
 }
    }
  return opic;
}

add(pavehexagonal(rotate(30)*hexa,3));

đź”—tiling-fig005

Figure tiling 005 Generated with Asymptote

Show tiling/fig0050.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling

size(10cm,0);

transform r60=rotate(60);
picture hexa;

pair A=(1,0);
pair B=r60*A, C=r60*B, D=r60*C, E=r60*D, F=r60*E;

real ad=30;
real tensio=.15;
path AB=A {dir(120-ad)} .. shift(tensio*dir(30))*midpoint(A--B)  .. B {dir(120+ad)};
path BC=reverse(rotate(240,B)*AB);
path CD=reverse(rotate(240,C)*BC);
path DE=reverse(rotate(240,D)*CD);
path EF=reverse(rotate(240,E)*DE);
path FA=reverse(rotate(240,F)*EF);

real lux=-.3, sq=sqrt(3)/2;
radialshade(hexa,AB--BC--CD--DE--EF--FA--cycle,
	    lightgrey,(lux*sq,lux/2),0,
	    grey,(lux*sq,lux/2),1+abs(lux));

//Circular paving with the unit hexagonal picture "hexa"
picture pavehexagonal(picture hexa, int depth=1)
{
  picture opic;
  pair center;
  real a,ap,r,rp,r_d=180/pi;

  add(opic, hexa);

  for(int j=0; j<depth; ++j)
    {
      for (int i=1; i<=6; ++i)
 {
   a=i*60-30;
   r=j*sqrt(3);
   center=r*(rotate(a)*(1,0));
   add(opic, shift(center)*hexa);
   rp=r;
   ap=0;
   for (real k=0; k<j-1; ++k)
     {
       r=sqrt((1.5*(j-1 - k))^2 + 3/4*(j+1 + k)^2);
       ap+=r_d*acos((rp^2 + r^2 - 3)/(2*r*rp));
       center=r*(rotate(a + ap)*(1,0));
       add(opic, shift(center)*hexa);
       rp=r;
     }
 }
    }
  return opic;
}

add(pavehexagonal(hexa,4));

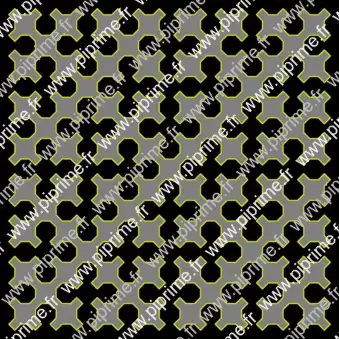
đź”—tiling-fig006

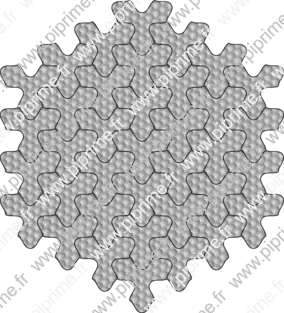
Figure tiling 006 Generated with Asymptote

Show tiling/fig0060.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling | #Clip

size(10cm,0);

transform r60=rotate(60);

pair A=(1,0);
pair B=r60*A, C=r60*B, D=r60*C, E=r60*D, F=r60*E;

real ad=30;
real tensio=.25;
path AB=A {dir(120-ad)} .. shift(tensio*dir(30))*midpoint(A--B)  .. B {dir(120+ad)};
path BC=reverse(rotate(240,B)*AB);
path CD=reverse(rotate(240,C)*BC);
path DE=reverse(rotate(240,D)*CD);
path EF=reverse(rotate(240,E)*DE);
path FA=reverse(rotate(240,F)*EF);
path pth1=AB--BC--CD--DE--EF--FA;

real tensio=.5;
path AB=A {dir(120-ad)} .. shift(tensio*dir(30))*midpoint(A--B)  .. B {dir(120+ad)};
path BC=reverse(rotate(240,B)*AB);
path CD=reverse(rotate(240,C)*BC);
path DE=reverse(rotate(240,D)*CD);
path EF=reverse(rotate(240,E)*DE);
path FA=reverse(rotate(240,F)*EF);
path pth2=AB--BC--CD--DE--EF--FA;


//Circular paving with the unit hexagonal picture "hexa"
picture pavehexagonal(picture hexa, int depth=1)
{
  picture opic;
  pair center;
  real a,ap,r,rp,r_d=180/pi;

  add(opic, hexa);

  for(int j=0; j<depth; ++j)
    {
      for (int i=1; i<=6; ++i)
 {
   a=i*60-30;
   r=j*sqrt(3);
   center=r*(rotate(a)*(1,0));
   add(opic, shift(center)*hexa);
   rp=r;
   ap=0;
   for (real k=0; k<j-1; ++k)
     {
       r=sqrt((1.5*(j-1 - k))^2 + 3/4*(j+1 + k)^2);
       ap+=r_d*acos((rp^2 + r^2 - 3)/(2*r*rp));
       center=r*(rotate(a + ap)*(1,0));
       add(opic, shift(center)*hexa);
       rp=r;
     }
 }
    }
  return opic;
}

picture hexa, hexat;

real lux=-.3, sq=sqrt(3);
radialshade(hexa,pth1--cycle,
	    lightgrey,(lux*sq/2,lux/2),0,
	    grey,(lux*sq/2,lux/2),1+abs(lux));


add(hexat,scale(1/(3*sq))*pavehexagonal(hexa,5));
clip(hexat,pth2--cycle);
draw(hexat,pth2);
add(pavehexagonal(hexat,4));

đź”—tiling-fig007

Figure tiling 007 Generated with Asymptote

Author: Guillaume Connan.

Show tiling/fig0070.asy on Github.
Generated with Asymptote 3.00-0.
Categories : Surveys | Tiling
Tags : #Tiling | #Transform | #Shipout | #Fill/Unfill

size(10cm,0);

void zigzag(int k)
{
  real t=180/k;
  pair o=(0,0), m=dir(t),
    n=rotate(180-2*t,m)*o,
    b=rotate(4*t-180,n)*m,
    c=rotate(180-6*t,b)*n,
    nn=reflect(o,b)*n;

  path lo=m--n--b--nn--cycle,
    p=o--m--n--b--c--cycle,
    pp=reflect(o,b)*p;

  for (int i=0; i <= k; ++i){
    filldraw(rotate(2*t*i,o)*p,.5*(red+blue));
    filldraw(rotate(2*t*i,o)*pp,0.25(red+blue));
    filldraw(rotate(2*t*i,o)*lo,(red+blue));
  }
}

zigzag(25);
shipout(bbox(3mm,2mm+miterjoin+black,FillDraw(0.5*blue)));

This page was generated by the Asymptote Exemples Builder
Last modified the 2025-04-20 12:26:19
©2011 Philippe Ivaldi

0%